Advertisements
Advertisements
प्रश्न
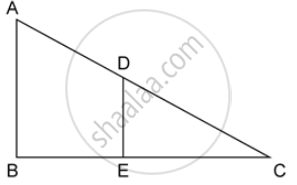
ABC is a right angled triangle with ∠ABC = 90°. D is any point on AB and DE is perpendicular to AC. Prove that :
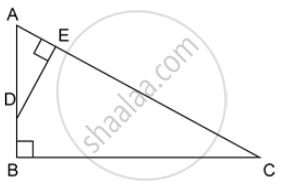
Find, area of ΔADE : area of quadrilateral BCED.
उत्तर
We need to find the area of ADE and quadrilateral BCED
Area of ΔADE = `1/2 xx AE XX DE = 1/2 xx 4 xx 5/3 = 10/3 cm^3`
Area of quad.BCED = Area of ΔABC Area of ΔADE
` = 1/2 xx BC xx AB - 10/3`
`= 1/2 xx 5 xx 12 - 10/3`
`= 30 - 10/3`
`= 80/3 cm^2`
Thus ratio of areas of ADE to quadrilateral BCED = `(10/3)/(80/3) = 1/8`
APPEARS IN
संबंधित प्रश्न
In the figure, given below, straight lines AB and CD intersect at P; and AC || BD. Prove that: If BD = 2.4 cm, AC = 3.6 cm, PD = 4.0 cm and PB = 3.2 cm; find the lengths of PA and PC.
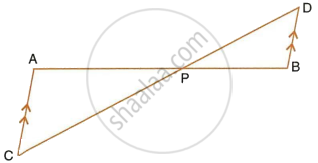
In the figure, given below, straight lines AB and CD intersect at P; and AC || BD. Prove that: ΔAPC and ΔBPD are similar.
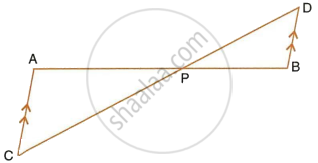
In a trapezium ABCD, side AB is parallel to side DC; and the diagonals AC and BD intersect each other at point P. Prove that : PA x PD = PB x PC.
In the given figure, AB || DC, BO = 6 cm and DQ = 8 cm; find: BP × DO.

In the following figure, XY is parallel to BC, AX = 9 cm, XB = 4.5 cm and BC = 18 cm.
Find : XY
Triangle ABC is similar to triangle PQR. If AD and PM are altitudes of the two triangles, prove that : `(AB)/(PQ) = (AD)/(PM)`.
In the given figure, AB and DE are perpendiculars to BC.
Prove that : ΔABC ~ ΔDEC
Triangles ABC and DEF are similar.
If area (ΔABC) = 16 cm, area (ΔDEF) = 25 cm2 and BC = 2.3 cm find EF.
In the given figure ΔABC and ΔAMP are right angled at B and M respectively.
Given AC = 10 cm, AP = 15 cm and PM = 12 cm.
(i) Prove ΔABC ∼ Δ AMP.
(ii) Find AB and BC.
Triangles ABC and DEF are similar.
If area (ΔABC) = 9 cm2, area (ΔDEF) = 64 cm2 and BC = 5·1 cm find AB.
