Advertisements
Advertisements
प्रश्न
Δ ABC ∼ Δ PQR. AD and PS are altitudes from A and P on sides BC and QR respectively. If AD : PS = 4 : 9 , find the ratio of the areas of Δ ABC and Δ PQR.
उत्तर
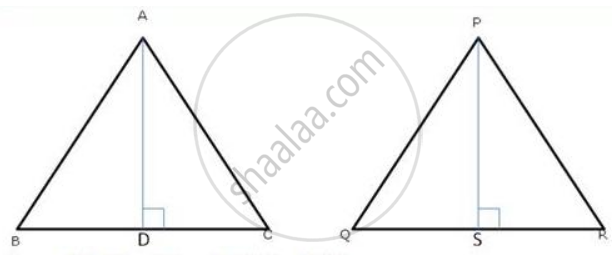
Given : AD : PS = 4 : 9 and Δ ABC ∼ Δ PQR
To Find : `("Ar" triangle "ABC")/("Ar" triangle "PQR")`
Sol : `("Ar" triangle "ABC")/("Ar" triangle "PQR") = ("AB"^2)/("PQ"^2)` .......(1)
[The ratio of area of two similar triangles is equal to the ratio of square of their corresponding sides]
In Δ BAD and Δ QPS
∠ B = ∠ Q (Δ ABC ∼ Δ PQR)
∠AOB = ∠PSQ (90° each)
Δ BAD ∼ Δ QPS (AA corollary)
`therefore "AB"/"PQ" = "AD"/"PS"` ........(2) (similar sides of similar triangles)
Using (1) and (2)
`("Ar" triangle "ABC")/("Ar" triangle "PQR") = "AD"^2/"PS"^2 = (4/9)^2 = 16/81`
Required ratio is 16 : 81.
APPEARS IN
संबंधित प्रश्न
In figure, if ∠A = ∠C, then prove that ∆AOB ~ ∆COD

State, true or false:
Two congruent polygons are necessarily similar.
The ratio between the corresponding sides of two similar triangles is 2 is to 5. Find the ratio between the areas of these triangles.
The given diagram shows two isosceles triangles which are similar. In the given diagram, PQ and BC are not parallel; PC = 4, AQ = 3, QB = 12, BC = 15 and AP = PQ.

Calculate:
- the length of AP,
- the ratio of the areas of triangle APQ and triangle ABC.
In the given figure, A – D – C and B – E – C seg DE || side AB If AD = 5, DC = 3, BC = 6.4 then Find BE.

Figure shows Δ KLM , P an T on KL and KM respectively such that∠ KLM =∠ KTP.
If `"KL"/"KT" = 9/5` , find `("Ar" triangle "KLM")/("Ar" triangle "KTP")`.

ABCD and PQRS are similar figures. AB= 12cm, BC=x cm, CD= 15 cm, AD= 10 cm, PQ= 8 cm, QR = 5 cm, RS = m cm and PS = n cm .Find the values of x, m and n.
A map is drawn to scale of 1:20000. Find: The distance on the map representing 4km
A flag pole 15 m high casts a shadow of 3 m at 10 a.m. The shadow cast by a building at the same time is 18.6 m. The height of the building is
In figure, if PQRS is a parallelogram and AB || PS, then prove that OC || SR.
