Advertisements
Advertisements
प्रश्न
Air is pushed into a soap bubble of radius r to double its radius. If the surface tension of the soap solution in S, the work done in the process is
पर्याय
8 π r2 S
12 π r2 S
16 π r2 S
24 π r2 S
उत्तर
\[\text{ No . of surfaces of a soap bubble } = 2\]
\[\text{ Increase in surface area }= 4\pi(2r )^2 - 4\pi(r )^2 = 12 \pi r^2 \]
\[\text{ Total increase in surface area }= 2 \times 12 \pi r^2 = 24 \pi r^2 \]
\[\text{ Work done = change in surface energy } \]
\[ = S \times 24 \pi r^2 = 24 \pi r^2 S\]
APPEARS IN
संबंधित प्रश्न
The surface tension of water at 0°C is 75.5 dyne/cm. Calculate surface tension of water at 25°C.
(α for water = 2.7×10-3/°C)
Derive an expression for excess pressure inside a drop of liquid.
In which of the following substances, surface tension increases with increase in temperature ?
- Copper
- Molten copper
- Iron
- Molten iron
Show that the surface tension of a liquid is numerically equal to the surface energy per unit
area.
A uniform vertical tube of circular cross section contains a liquid. The contact angle is 90°. Consider a diameter of the tube lying in the surface of the liquid. The surface to the right of this diameter pulls the surface on the left of it. What keeps the surface on the left in equilibrium?
If more air is pushed in a soap bubble, the pressure in it
The excess pressure inside a soap bubble is twice the excess pressure inside a second soap bubble. The volume of the first bubble is n times the volume of the second where n is
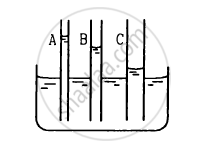
The capillaries shown in figure have inner radii 0.5 mm, 1.0 mm and 1.5 mm respectively. The liquid in the beaker is water. Find the heights of water level in the capillaries. The surface tension of water is 7.5 × 10−2 N m−1.
A hollow spherical body of inner and outer radii 6 cm and 8 cm respectively floats half-submerged in water. Find the density of the material of the sphere.
Derive an expression for capillary rise for a liquid having a concave meniscus.
What do you mean by capillarity or capillary action?
A drop of oil placed on the surface of water spreads out. But a drop of water place on oil contracts to a spherical shape. Why?
If the surface tension of a soap solution is 3 × 10-2 N/m then the work done in forming a soap film of 20 cm × 5 cm will be ______.
The wear and tear in the machine part is due to ______.
What is surface tension? Explain the applications of surface tension.
Two mercury droplets of radii 0.1 cm. and 0.2 cm. collapse into one single drop. What amount of energy is released? The surface tension of mercury T = 435.5 × 10–3 Nm–1.
This model of the atmosphere works for relatively small distances. Identify the underlying assumption that limits the model.
A coaxial cylinder made of glass is immersed in liquid of surface tension ' S'. Radius of inner and outer surface of cylinder are R1 and R2 respectively. Height till which liquid will rise is (Density of liquid is p):
Work done to blow a bubble of volume V is W. The work done in blowing a bubble of volume 2V will be ______.
