Advertisements
Advertisements
प्रश्न
An inductor of inductance 2.00 H is joined in series with a resistor of resistance 200 Ω and a battery of emf 2.00 V. At t = 10 ms, find (a) the current in the circuit, (b) the power delivered by the battery, (c) the power dissipated in heating the resistor and (d) the rate at which energy is being stored in magnetic field.
उत्तर
Given:-
Inductance of the inductor, L = 2 H
Resistance of the resistor connected to the inductor, R = 200 Ω,
Emf of the battery connected, E = 2 V
(a) The current in the LR circuit after t seconds after connecting the battery is given by
i = i0(1 − e−t/τ)
Here,
i0 = Steady state value of current
`i_0 = E/R=2/200A`
At time t = 10 ms, the current is given by
\[i= \frac{2}{200}(1 - e^{- 10 \times {10}^{- 3} \times 200/2} )\]
i = 0.01(1 − e−1)
i = 0.01(1 − 0.3678)
i = 0.01 × 0.632 = 6.3 mA
(b) The power delivered by the battery is given by
P = Vi
P = Ei0(1 − e−t/τ)
\[P = \frac{E^2}{R}(1 - e^{- t/\tau} )\]
\[P = \frac{2 \times 2}{200}(1 - e^{10 \times {10}^{- 3} \times 200/2} )\]
P = 0.02(1 − e−1)
P = 0.01264 = 12.6 mW
(c) The power dissipated in the resistor is given by
P1 = i2R
P1 = [i0(1 − e−t/τ)]2 R
P1 = (6.3 mA)2 × 200
P1 = 6.3 × 6.3 × 200 × 10−5
P1 = 79.38 × 10−4
P1 = 7.938 × 10−3 = 8 mW
(d) The rate at which the energy is stored in the magnetic field can be calculated as:-
\[W =\frac{1}{2}L i^2\]
\[W= \frac{L}{2} {i_0}^2 (1 - e^{- t/\tau} )^2\]
W = 2 × 10−2(0.225)
W = 0.455 × 10−2
W = 4.6 × 10−3
W = 4.6 mW
APPEARS IN
संबंधित प्रश्न
In a series LCR circuit, VL = VC ≠ VR. What is the value of power factor?
A voltage V = V0 sin ωt is applied to a series LCR circuit. Derive the expression for the average power dissipated over a cycle. Under what condition (i) no power is dissipated even though the current flows through the circuit, (ii) maximum power is dissipated in the circuit?
(i) Find the value of the phase difference between the current and the voltage in the series LCR circuit shown below. Which one leads in phase : current or voltage ?
(ii) Without making any other change, find the value of the additional capacitor C1, to be connected in parallel with the capacitor C, in order to make the power factor of the circuit unity.
A series LCR circuit is connected to a source having voltage v = vm sin ωt. Derive the expression for the instantaneous current I and its phase relationship to the applied voltage.
Obtain the condition for resonance to occur. Define ‘power factor’. State the conditions under which it is (i) maximum and (ii) minimum.
A coil of resistance 40 Ω is connected across a 4.0 V battery. 0.10 s after the battery is connected, the current in the coil is 63 mA. Find the inductance of the coil.
The potential difference across the resistor is 160V and that across the inductor is 120V. Find the effective value of the applied voltage. If the effective current in the circuit be 1.0 A, calculate the total impedance of the circuit.
What will be the potential difference in the circuit when direct current is passed through the circuit?
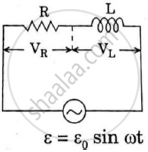
Using the phasor diagram, derive the expression for the current flowing in an ideal inductor connected to an a.c. source of voltage, v= vo sin ωt. Hence plot graphs showing the variation of (i) applied voltage and (ii) the current as a function of ωt.
Derive an expression for the average power dissipated in a series LCR circuit.
Choose the correct answer from given options
The selectivity of a series LCR a.c. circuit is large, when
In an LCR series a.c. circuit, the voltage across each of the components, L, C and R is 50V. The voltage across the LC combination will be ______.
A coil of 40 henry inductance is connected in series with a resistance of 8 ohm and the combination is joined to the terminals of a 2 volt battery. The time constant of the circuit is ______.
In series LCR circuit, the phase angle between supply voltage and current is ______.
At resonance frequency the impedance in series LCR circuit is ______.
In series LCR AC-circuit, the phase angle between current and voltage is
In an LCR circuit having L = 8 henery. C = 0.5 µF and R = 100 ohm in series, the resonance frequency in radian/sec is
Which of the following components of an LCR circuit, with a.c. supply, dissipates energy?
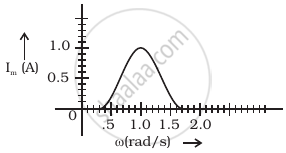
In series LCR circuit, the plot of Imax vs ω is shown in figure. Find the bandwidth and mark in the figure.
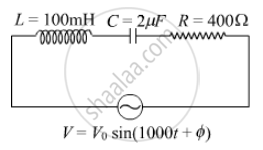
A series LCR circuit containing a resistance of 120 Ω has angular resonance frequency 4 × 105 rad s-1. At resonance the voltage across resistance and inductance are 60 V and 40 V respectively. At what frequency the current in the circuit lags the voltage by 45°. Give answer in ______ × 105 rad s-1.
Draw the impedance triangle for a series LCR AC circuit and write the expressions for the impedance and the phase difference between the emf and the current.
