Advertisements
Advertisements
प्रश्न
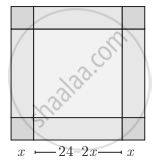
An open box is to be made from a square piece of material, 24 cm on a side, by cutting equal square from the corner and turning up the side as shown. Express the volume V of the box as a function of x
उत्तर
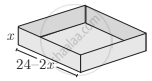
After cutting squares we will get a cuboid,
length of the cuboid (l) = 24 – 2x
breadth of the cuboid (b) = 24 – 2x
height of the cuboid (h) = 2x
Volume of the box = Volume of the cuboid
V = (24 – 2x)(24 – 2x) (x)
= (24 – 2x)2 (x)
= (576 + 4x2 – 96x) x
= 576x + 4x3 – 96x2
V = 4x3 – 96x2 + 576x
V(x) = 4x3 – 96x2 + 576x
APPEARS IN
संबंधित प्रश्न
If f is a real function satisfying \[f\left( x + \frac{1}{x} \right) = x^2 + \frac{1}{x^2}\]
for all x ∈ R − {0}, then write the expression for f(x).
Let A and B be two sets such that n(A) = p and n(B) = q, write the number of functions from A to B.
Let A = {x ∈ R : x ≠ 0, −4 ≤ x ≤ 4} and f : A ∈ R be defined by \[f\left( x \right) = \frac{\left| x \right|}{x}\] for x ∈ A. Then th (is
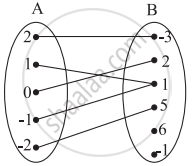
Check if the following relation is a function.
Find the domain and range of the following function.
g(x) = `(x + 4)/(x - 2)`
Express the area A of circle as a function of its circumference C.
If `log((x + y)/3) = 1/2 log x + 1/2 logy`, show that `x/y + y/x` = 7
A function f is defined by f(x) = 2x – 3 find x such that f(x) = x
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find (f + g)(x)
Let f(x) = `sqrt(1 + x^2)`, then ______.
