Advertisements
Advertisements
प्रश्न
Angles of a triangle are in the ratio 2 : 4 : 3. The smallest angle of the triangle is ______.
पर्याय
60°
40°
80°
20°
उत्तर
Angles of a triangle are in the ratio 2 : 4 : 3. The smallest angle of the triangle is 40°.
Explanation:
Given, the ratio of angles of a triangle is 2 : 4 : 3.
Let the angles of a triangle be ∠A, ∠B and ∠C.
∠A = 2x, ∠B = 4x and ∠C = 3x ,
∠A + ∠B + ∠C = 180° ...[Sum of all the angles of a triangle is 180°]
2x + 4x + 3x = 180°
9x = 180°
x = `(180^circ)/9` = 20°
∠A = 2x = 2 × 20° = 40°
∠B = 4x = 4 × 20° = 80°
∠C = 3x = 3 × 20° = 60°
Hence, the smallest angle of a triangle is 40°.
APPEARS IN
संबंधित प्रश्न
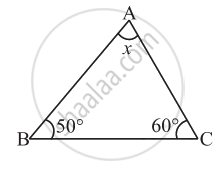
Find the value of the unknown x in the following diagram:
Find the value of the unknown x and y in the following diagram:

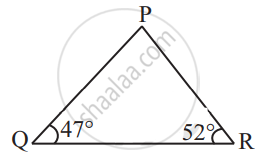
In the given figure find m∠P.
In the following triangle, find the value of x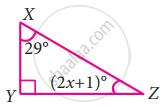
How many triangles can be drawn having its angles as 53°, 64° and 63°? Give reason for your answer.
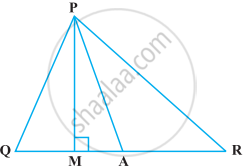
In the following figure, ∠Q > ∠R, PA is the bisector of ∠QPR and PM ⊥ QR. Prove that ∠APM = `1/2` (∠Q – ∠R).
In the given figure, find the values of a, b and c
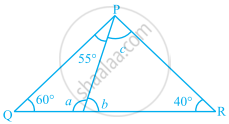
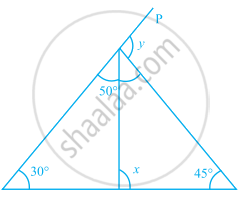
Find the values of x and y in the given figure.
In ΔPQR, if 3∠P = 4∠Q = 6∠R, calculate the angles of the triangle.
In the given figure, if ST = SU, then find the values of x and y.

