Advertisements
Advertisements
प्रश्न
Complete of the following, so as to make a true statement:
A point is in the interior of a convex quadrilateral, if it is in the ..... of its two opposite angles.
उत्तर
A point is in the interior of a convex quadrilateral, if it is in the interiors of its two opposite angles.
APPEARS IN
संबंधित प्रश्न
Complete of the following, so as to make a true statement:
A quadrilateral has ...... angles.
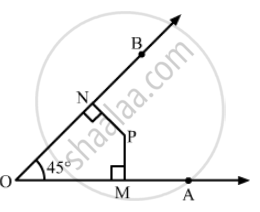
In Fig. 16.20, find the measure of ∠MPN.
In a convex hexagon, prove that the sum of all interior angle is equal to twice the sum of its exterior angles formed by producing the sides in the same order.
In a pentagon ABCDE, AB || ED and ∠B = 140°, ∠C = 2x° and ∠D = 3x°. Find ∠C and ∠D
ΔPQR and ΔSQR are on the same base QR with P and S on opposite sides of line QR, such that area of ΔPQR is equal to the area of ΔSQR. Show that QR bisects PS.
A quadrilateral can have all four angles as obtuse.
Construct a quadrilateral NEWS in which NE = 7 cm, EW = 6 cm, ∠N = 60°, ∠E = 110° and ∠S = 85°.
If the sum of two angles is greater than 180°, then which of the following is not possible for the two angles?
In the given figure.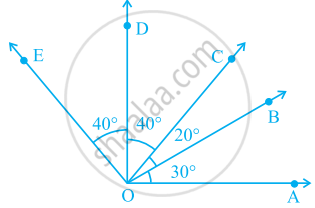
∠AOE is a/an ______ angle
Draw a rough sketch of a quadrilateral PQRS. Draw its diagonals. Name them. Is the meeting point of the diagonals in the interior or exterior of the quadrilateral?