Advertisements
Advertisements
प्रश्न
Consider the given parallelogram. Find the values of the unknowns x, y, z.

उत्तर १
x + 100° = 180° (Adjacent angles are supplementary)
x = 80°
z = x = 80º(Opposite angles are equal)
y = 100° (Opposite angles are equal)
उत्तर २
ABCD is a parallelogram in which ∠B = 100° (given)
∴ ∠A + ∠B = 180° ...[∵ Sum of adjacent angles is 180°]
⇒ z + 100° = 180°
⇒ z = 180° − 100° = 80°
Also ∠B = ∠D and ∠A = ∠C ...[∵ Opposite angles are equal]
∴ ∠B = 100° = ∠D = y and ∠A = z = 80° = ∠C = x
∴ x = 80°, y = 100°, z = 80°
APPEARS IN
संबंधित प्रश्न
In the given figure, G is the point of concurrence of medians of ΔDEF. Take point H on ray DG such that D-G-H and DG = GH, then prove that `square`GEHF is a parallelogram.

Given: Parallelogram ABCD in which diagonals AC and BD intersect at M.
Prove: M is the mid-point of LN.
ABCD is a parallelogram. What kind of quadrilateral is it if : AC = BD and AC is perpendicular to BD?
ABCD is a parallelogram. What kind of quadrilateral is it if: AC is perpendicular to BD but is not equal to it?
In parallelogram ABCD, E is the mid-point of AD and F is the mid-point of BC. Prove that BFDE is a parallelogram.
In the given figure, AB || EC, AB = AC and AE bisects ∠DAC. Prove that:
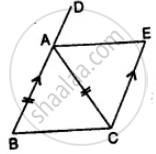
- ∠EAC = ∠ACB
- ABCE is a parallelogram.
If two adjacent angles of a parallelogram are (5x – 5)° and (10x + 35)°, then the ratio of these angles is ______.
The angle between the two altitudes of a parallelogram through the same vertex of an obtuse angle of the parallelogram is 30°. The measure of the obtuse angle is ______.
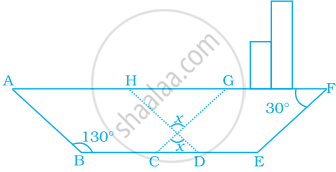
In the following figure of a ship, ABDH and CEFG are two parallelograms. Find the value of x.
ABCD is a parallelogram. The bisector of angle A intersects CD at X and bisector of angle C intersects AB at Y. Is AXCY a parallelogram? Give reason.
