Advertisements
Advertisements
प्रश्न
Draw circle with diameter: 8.4 cm
In above case, measure the length of the radius of the circle drawn.
उत्तर
AB is the diameter of circle
i.e. AB = 8.4 cm
and OA is the radius of a circle
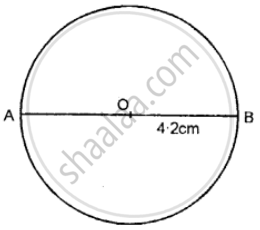
`= 1/2 "of diameter" = 8.4/2 = 4.2` cm
i.e., OA = OB = 4.2 cm.
APPEARS IN
संबंधित प्रश्न
In the below fig. O is the centre of the circle. If ∠APB = 50°, find ∠AOB and ∠OAB.

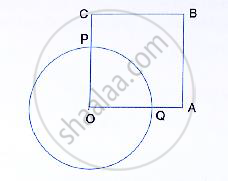
In the following figure, OABC is a square. A circle is drawn with O as centre which meets OC
at P and OA at Q. Prove that:
(i) ΔOPA ≅ ΔOQC, (ii) ΔBPC ≅ ΔBQA.
Two concentric circles are of diameters 30 cm and 18 cm. Find the length of the chord of the larger circle which touches the smaller circle.
AB is a chord of a circle with centre O , AOC is a diameter and AT is the tangent at A as shown in Fig . 10.70. Prove that \[\angle\]BAT = \[\angle\] ACB.
The point of concurrence of all angle bisectors of a triangle is called the ______.
The longest chord of a circle is __________
Given: A circle inscribed in a right angled ΔABC. If ∠ACB = 90° and the radius of the circle is r.
To prove: 2r = a + b – c
If d1, d2 (d2 > d1) be the diameters of two concentric circles and c be the length of a chord of a circle which is tangent to the other circle, then ______
In the following figure, if AOB is a diameter of the circle and AC = BC, then ∠CAB is equal to ______.

In the following figure, BC is a diameter of the circle and ∠BAO = 60º. Then ∠ADC is equal to ______.