Advertisements
Advertisements
प्रश्न
Evaluate the following limits:
`lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5)`
उत्तर
`lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5) = lim_(x -> 5) ((x - 1)^(1/2) - (4)^(1/2))/((x - 1) - 4)`
Put x – 1 = y
When x → 1
⇒ y → 5 – 1 = 4
∴ `lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5) = lim_(y -> 4) (^(1/2) - (4)^(1/2))/(y - 4)`
`lim_(x -> "a") (x^"n" - "a"^"n")/(x - "a") = "n" * "a"^("n" - 1)`
= `1/2(4)^(1/2 - 1)`
= `1/2 xx (4)^(- 1/2)`
= `1/2 xx 1/((4)^(1/2))`
= `1/2 xx 1/2`
`lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5) = 1/4`
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit:
`lim_(z -> -5)[((1/z + 1/5))/(z + 5)]`
Evaluate the following limit:
`lim_(x -> 3)[sqrt(2x + 6)/x]`
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 2) f(x)` where `f(x) = {{:(4 - x",", x ≠ 2),(0",", x = 2):}`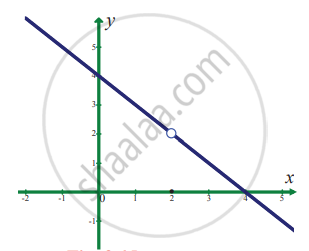
Sketch the graph of a function f that satisfies the given value:
f(0) is undefined
`lim_(x -> 0) f(x)` = 4
f(2) = 6
`lim_(x -> 2) f(x)` = 3
Evaluate the following limits:
`lim_("h" -> 0) (sqrt(x + "h") - sqrt(x))/"h", x > 0`
Evaluate the following limits:
`lim_(x -> 1) (sqrt(x) - x^2)/(1 - sqrt(x))`
Evaluate the following limits:
`lim_(x - 0) (sqrt(1 + x^2) - 1)/x`
Evaluate the following limits:
`lim_(x -> 3) (x^2 - 9)/(x^2(x^2 - 6x + 9))`
Evaluate the following limits:
`lim_(x -> oo) 3/(x - 2) - (2x + 11)/(x^2 + x - 6)`
Evaluate the following limits:
`lim_(x -> oo) (1 + x - 3x^3)/(1 + x^2 +3x^3)`
Evaluate the following limits:
`lim_(x -> 0)(1 + x)^(1/(3x))`
Evaluate the following limits:
`lim_(x -> oo)(1 + "k"/x)^("m"/x)`
Evaluate the following limits:
`lim_(x -> 0) (sinalphax)/(sinbetax)`
Evaluate the following limits:
`lim_(x -> 0) (tan 2x)/(sin 5x)`
Evaluate the following limits:
`lim_(x -> 0) (2^x - 3^x)/x`
Evaluate the following limits:
`lim_(x -> 0) (tan x - sin x)/x^3`
Choose the correct alternative:
`lim_(x -> 0) sqrt(1 - cos 2x)/x`
If `lim_(x->1)(x^5-1)/(x-1)=lim_(x->k)(x^4-k^4)/(x^3-k^3),` then k = ______.
`lim_(x -> 0) (sin 4x + sin 2x)/(sin5x - sin3x)` = ______.
