Advertisements
Advertisements
Question
Evaluate the following limits:
`lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5)`
Solution
`lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5) = lim_(x -> 5) ((x - 1)^(1/2) - (4)^(1/2))/((x - 1) - 4)`
Put x – 1 = y
When x → 1
⇒ y → 5 – 1 = 4
∴ `lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5) = lim_(y -> 4) (^(1/2) - (4)^(1/2))/(y - 4)`
`lim_(x -> "a") (x^"n" - "a"^"n")/(x - "a") = "n" * "a"^("n" - 1)`
= `1/2(4)^(1/2 - 1)`
= `1/2 xx (4)^(- 1/2)`
= `1/2 xx 1/((4)^(1/2))`
= `1/2 xx 1/2`
`lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5) = 1/4`
APPEARS IN
RELATED QUESTIONS
Evaluate the following limit :
`lim_(x -> 1)[(x + x^2 + x^3 + ......... + x^"n" - "n")/(x - 1)]`
Evaluate the following limit :
`lim_(x -> 7)[((root(3)(x) - root(3)(7))(root(3)(x) + root(3)(7)))/(x - 7)]`
Evaluate the following limit :
`lim_(x -> 7) [(x^3 - 343)/(sqrt(x) - sqrt(7))]`
Evaluate the following :
Find the limit of the function, if it exists, at x = 1
f(x) = `{(7 - 4x, "for", x < 1),(x^2 + 2, "for", x ≥ 1):}`
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 2) (x - 2)/(x^2 - 4)`
| x | 1.9 | 1.99 | 1.999 | 2.001 | 2.01 | 2.1 |
| f(x) | 0.25641 | 0.25062 | 0.250062 | 0.24993 | 0.24937 | 0.24390 |
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 3) (4 - x)`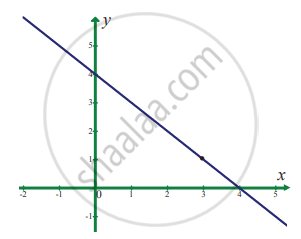
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 5) |x - 5|/(x - 5)`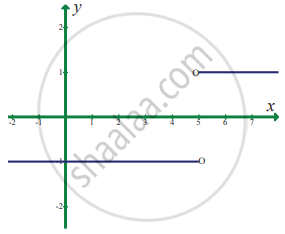
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 1) sin pi x`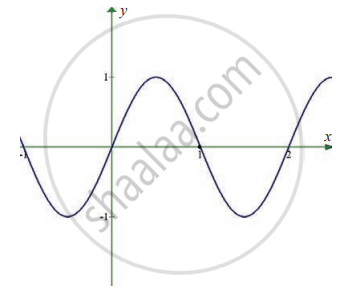
Sketch the graph of a function f that satisfies the given value:
f(0) is undefined
`lim_(x -> 0) f(x)` = 4
f(2) = 6
`lim_(x -> 2) f(x)` = 3
Evaluate the following limits:
`lim_(x -> 1) (root(3)(7 + x^3) - sqrt(3 + x^2))/(x - 1)`
Evaluate the following limits:
`lim_(x -> 0) (sqrt(1 - x) - 1)/x^2`
Evaluate the following limits:
`lim_(x -> oo) 3/(x - 2) - (2x + 11)/(x^2 + x - 6)`
Evaluate the following limits:
`lim_(x -> oo) ((2x^2 + 3)/(2x^2 + 5))^(8x^2 + 3)`
Evaluate the following limits:
`lim_(x -> oo) x [3^(1/x) + 1 - cos(1/x) - "e"^(1/x)]`
Choose the correct alternative:
`lim_(x -> oo) sinx/x`
Choose the correct alternative:
`lim_(x -> 0) sqrt(1 - cos 2x)/x`
Choose the correct alternative:
`lim_(theta -> 0) (sinsqrt(theta))/(sqrt(sin theta)`
Choose the correct alternative:
If `lim_(x -> 0) (sin "p"x)/(tan 3x)` = 4, then the value of p is
Choose the correct alternative:
`lim_(x -> 0) ("e"^tanx - "e"^x)/(tan x - x)` =
`lim_(x→-1) (x^3 - 2x - 1)/(x^5 - 2x - 1)` = ______.
