Advertisements
Advertisements
प्रश्न
Fill in the blank to make the following statement true:
A triangles cannot have more than ......obtuse angles.
उत्तर
A triangle cannot have more than one obtuse angle
As the sum of all the angles of a triangle is 180°. So, if the triangle has more than one obtuse angle the sum would exceed 180°.
APPEARS IN
संबंधित प्रश्न
Can a triangle have All angles equal to 60°? Justify your answer in case.
Mark the correct alternative in each of the following:
If all the three angles of a triangle are equal, then each one of them is equal to
State, if the triangle is possible with the following angles :
125°, 40°, and 15°
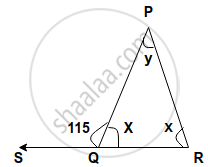
Find the unknown marked angles in the given figure:

Find the unknown marked angles in the given figure:
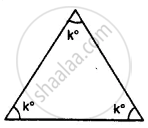
Find, giving a reason, the unknown marked angles, in a triangle drawn below:
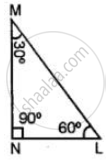
Classify the following triangle according to sides:

Classify the following triangle according to angle:
In the following figure, AD is the bisector of ∠BAC. Prove that AB > BD.

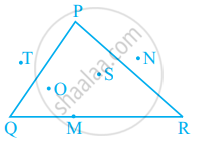
In figure, points lying in the interior of the triangle PQR are ______, that in the exterior are ______ and that on the triangle itself are ______.