Advertisements
Advertisements
प्रश्न
Find the equation of the parabola if
the focus is at (0, −3) and the vertex is at (0, 0)
उत्तर
In a parabola, the vertex is the mid-point of the focus and the point of intersection of the axis and the directrix.
Let (x1, y1) be the coordinates of the point of intersection of thIt is given that the vertex and the focus of a parabola are (0, 0) and (0, −3), respectively.
Thus, the slope of the axis of the parabola cannot be defined.
Slope of the directrix = 0
Let the directrix intersect the axis at K (r, s). e axis and directrix.
∴ \[\frac{r + 0}{2} = 0, \frac{s - 3}{2} = 0\]
\[ \Rightarrow r = 0, s = 3\]
∴ Required equation of directrix:
\[y = 3\]
Let P (x, y) be any point on the parabola whose focus is S (0, −3) and the directrix is \[y = 3\]
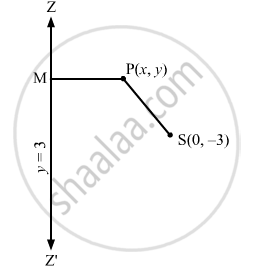 Draw PM perpendicular to \[y = 3\]
Draw PM perpendicular to \[y = 3\]
Then, we have:
\[SP = PM\]
\[ \Rightarrow S P^2 = P M^2 \]
\[ \Rightarrow \left( x - 0 \right)^2 + \left( y + 3 \right)^2 = \left( \frac{y - 3}{\sqrt{1}} \right)^2 \]
\[ \Rightarrow x^2 + y^2 + 6y + 9 = y^2 - 6y + 9\]
\[ \Rightarrow x^2 = - 12y\]
APPEARS IN
संबंधित प्रश्न
Find the equation of the parabola that satisfies the following condition:
Focus (0, –3); directrix y = 3
Find the equation of the parabola that satisfies the following condition:
Vertex (0, 0) focus (–2, 0)
If a parabolic reflector is 20 cm in diameter and 5 cm deep, find the focus.
Find the equation of the parabola whose:
focus is (3, 0) and the directrix is 3x + 4y = 1
Find the equation of the parabola whose:
focus is (2, 3) and the directrix x − 4y + 3 = 0.
Find the equation of the parabola whose focus is the point (2, 3) and directrix is the line x − 4y + 3 = 0. Also, find the length of its latus-rectum.
Find the equation of the parabola if
the focus is at (−6, −6) and the vertex is at (−2, 2)
Find the equation of the parabola if the focus is at (a, 0) and the vertex is at (a', 0)
Find the equation of the parabola if the focus is at (0, 0) and vertex is at the intersection of the lines x + y = 1 and x − y = 3.
At what point of the parabola x2 = 9y is the abscissa three times that of ordinate?
Find the equation of a parabola with vertex at the origin, the axis along x-axis and passing through (2, 3).
Find the equation of a parabola with vertex at the origin and the directrix, y = 2.
Find the equation of the parabola whose focus is (5, 2) and having vertex at (3, 2).
Find the equations of the lines joining the vertex of the parabola y2 = 6x to the point on it which have abscissa 24.
If the line y = mx + 1 is tangent to the parabola y2 = 4x, then find the value of m.
Write the equation of the directrix of the parabola x2 − 4x − 8y + 12 = 0.
Write the equation of the parabola with focus (0, 0) and directrix x + y − 4 = 0.
PSQ is a focal chord of the parabola y2 = 8x. If SP = 6, then write SQ.
Write the equation of the parabola whose vertex is at (−3,0) and the directrix is x + 5 = 0.
The equation 16x2 + y2 + 8xy − 74x − 78y + 212 = 0 represents
The locus of the points of trisection of the double ordinates of a parabola is a
The equation of the directrix of the parabola whose vertex and focus are (1, 4) and (2, 6) respectively is
The equation of the parabola whose focus is (1, −1) and the directrix is x + y + 7 = 0 is
The equation of the parabola whose focus is the point (2, 3) and directrix is the line x – 4y + 3 = 0 is ______.
Find the coordinates of a point on the parabola y2 = 8x whose focal distance is 4.
Find the equation of the following parabolas:
Vertex at (0, 4), focus at (0, 2)
Find the equation of the following parabolas:
Focus at (–1, –2), directrix x – 2y + 3 = 0
Find the equation of the set of all points the sum of whose distances from the points (3, 0) and (9, 0) is 12.
Find the equation of the set of all points whose distance from (0, 4) are `2/3` of their distance from the line y = 9.
The equation of the parabola having focus at (–1, –2) and the directrix x – 2y + 3 = 0 is ______.
If the vertex of the parabola is the point (–3, 0) and the directrix is the line x + 5 = 0, then its equation is ______.
The equation of the ellipse whose focus is (1, –1), the directrix the line x – y – 3 = 0 and eccentricity `1/2` is ______.
