Advertisements
Advertisements
प्रश्न
Find the measure of each exterior angle of an equilateral triangle.
उत्तर
Given to find the measure of each exterior angle of an equilateral triangle consider an
equilateral triangle ABC.
We know that for an equilateral triangle
AB = BC = CA and
`∠ABC =∠BCA =∠CAB=180^@/3=60^@` .......1
Now,
Extend side BC to D, CA to E and AB to F.
Here
BCD is a straight line segment
`⇒∠BCD = Straight angle 180^@`
`∠BCA+∠ACD=180^@`
`⇒60^@+∠ACD=180^@`
Similarly, we can find ∠FAB and ∠FBC also as `120^@` because ABC is an equilateral
triangle
`∴∠ACD =∠EAB =∠FBC =120^@`
Hence, the median of each exterior angle of an equilateral triangle is `120^@`
APPEARS IN
संबंधित प्रश्न
Show that the angles of an equilateral triangle are 60° each.
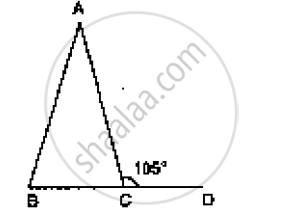
In Figure AB = AC and ∠ACD =105°, find ∠BAC.
Angles A, B, C of a triangle ABC are equal to each other. Prove that ΔABC is equilateral.
ABC is a triangle in which BE and CF are, respectively, the perpendiculars to the sides AC and AB. If BE = CF, prove that ΔABC is isosceles
Fill the blank in the following so that the following statement is true.
In an equilateral triangle all angles are .....
Which of the following statements are true (T) and which are false (F)?
Sum of any two sides of a triangle is greater than twice the median drawn to the third side.
Fill in the blank to make the following statement true.
Difference of any two sides of a triangle is........ than the third side.
In ΔABC, if ∠A = 100°, AD bisects ∠A and AD ⊥ BC. Then, ∠B =
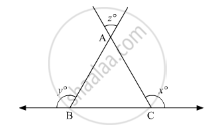
In the given figure, what is z in terms of x and y?
If the bisectors of the acute angles of a right triangle meet at O, then the angle at Obetween the two bisectors is
