Advertisements
Advertisements
प्रश्न
In ΔABC, if ∠A = 100°, AD bisects ∠A and AD ⊥ BC. Then, ∠B =
पर्याय
50°
90°
40°
100°
उत्तर
In the given ΔABC, ∠A= 100°, AD bisects ∠Aand AD ⊥ BC.
Here, we need to find ∠B.
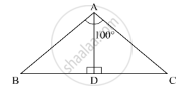
As, AD bisects∠A,
We get,
∠BAD = ∠DAC
100 = 2∠BAD
∠BAD = 50°
Now, according to angle sum property of the triangle
In ΔABD
∠A + ∠B + ∠D = 180°
50° + ∠B + 90° = 180°
140° + ∠B = 180°
∠B = 180° - 140°
= 40°
Hence, ∠B = 40°
APPEARS IN
संबंधित प्रश्न
If the base of an isosceles triangle is produced on both sides, prove that the exterior angles so formed are equal to each other.
Which of the following statements are true (T) and which are false (F):
The two altitudes corresponding to two equal sides of a triangle need not be equal.
Fill the blank in the following so that the following statement is true.
Sides opposite to equal angles of a triangle are ......
Fill the blank in the following so that the following statement is true.
In an equilateral triangle all angles are .....
In ΔABC, side AB is produced to D so that BD = BC. If ∠B = 60° and ∠A = 70°, prove that: (i) AD > CD (ii) AD > AC
O is any point in the interior of ΔABC. Prove that
(i) AB + AC > OB + OC
(ii) AB + BC + CA > OA + QB + OC
(iii) OA + OB + OC >` 1/2`(AB + BC + CA)
Which of the following statements are true (T) and which are false (F)?
Of all the line segments that can be drawn from a point to a line not containing it, the perpendicular line segment is the shortest one.
In the given figure, the sides BC, CA and AB of a Δ ABC have been produced to D, E and F respectively. If ∠ACD = 105° and ∠EAF = 45°, find all the angles of the Δ ABC.
CDE is an equilateral triangle formed on a side CD of a square ABCD (Figure). Show that ∆ADE ≅ ∆BCE.
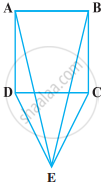
Show that in a quadrilateral ABCD, AB + BC + CD + DA > AC + BD
