Advertisements
Advertisements
प्रश्न
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
f (x) = (x −1)2 + 3, x ∈[−3, 1]
उत्तर
Given function f(x) = (x - 1)2 + 3 in the interval [-3, 1]
∴ f'(x) = 2(x - 1)
For critical points, let f' (x) = 0
If f'(x) = 0, then 2(x - 1) = 0,
⇒ x = 1 ∈ [-3, 1]
At, x = 1 f(1) = (1 - 1)2 + 3
= 0 + 3
= 3
At, x = -3 f(-3)
= (-3, -1)2 + 3
= 16 + 3 = 19
∴ Absolute maximum value of f(x) 19 at x = -3
Absolute minimum value of f(x) = 3 at x = 1.
APPEARS IN
संबंधित प्रश्न
Show that the height of the cylinder of maximum volume, that can be inscribed in a sphere of radius R is `(2R)/sqrt3.` Also, find the maximum volume.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`g(x) = x/2 + 2/x, x > 0`
Find the local maxima and local minima, if any, of the following functions. Find also the local maximum and the local minimum values, as the case may be:
`f(x) = xsqrt(1-x), x > 0`
At what points in the interval [0, 2π], does the function sin 2x attain its maximum value?
What is the maximum value of the function sin x + cos x?
Show that the right circular cylinder of given surface and maximum volume is such that is heights is equal to the diameter of the base.
Show that the right circular cone of least curved surface and given volume has an altitude equal to `sqrt2` time the radius of the base.
A rod of 108 meters long is bent to form a rectangle. Find its dimensions if the area is maximum. Let x be the length and y be the breadth of the rectangle.
The volume of a closed rectangular metal box with a square base is 4096 cm3. The cost of polishing the outer surface of the box is Rs. 4 per cm2. Find the dimensions of the box for the minimum cost of polishing it.
Find the maximum and minimum of the following functions : f(x) = 2x3 – 21x2 + 36x – 20
Divide the number 20 into two parts such that sum of their squares is minimum.
Solve the following:
A wire of length l is cut into two parts. One part is bent into a circle and the other into a square. Show that the sum of the areas of the circle and the square is the least, if the radius of the circle is half of the side of the square.
Divide the number 20 into two parts such that their product is maximum.
The total cost of producing x units is ₹ (x2 + 60x + 50) and the price is ₹ (180 − x) per unit. For what units is the profit maximum?
If f(x) = x.log.x then its maximum value is ______.
A rectangular sheet of paper has it area 24 sq. Meters. The margin at the top and the bottom are 75 cm each and the sides 50 cm each. What are the dimensions of the paper if the area of the printed space is maximum?
The minimum value of Z = 5x + 8y subject to x + y ≥ 5, 0 ≤ x ≤ 4, y ≥ 2, x ≥ 0, y ≥ 0 is ____________.
The maximum value of function x3 - 15x2 + 72x + 19 in the interval [1, 10] is ______.
The two parts of 120 for which the sum of double of first and square of second part is minimum, are ______.
The sum of two non-zero numbers is 6. The minimum value of the sum of their reciprocals is ______.
A metal box with a square base and vertical sides is to contain 1024 cm3. The material for the top and bottom costs Rs 5/cm2 and the material for the sides costs Rs 2.50/cm2. Find the least cost of the box.
The function f(x) = 2x3 – 3x2 – 12x + 4, has ______.
The maximum value of sin x . cos x is ______.
If y `= "ax - b"/(("x" - 1)("x" - 4))` has a turning point P(2, -1), then find the value of a and b respectively.
The function f(x) = x5 - 5x4 + 5x3 - 1 has ____________.
The area of a right-angled triangle of the given hypotenuse is maximum when the triangle is ____________.
Find the area of the largest isosceles triangle having a perimeter of 18 meters.
Let f(x) = 1 + 2x2 + 22x4 + …… + 210x20. Then f (x) has ____________.
The function `f(x) = x^3 - 6x^2 + 9x + 25` has
Divide 20 into two ports, so that their product is maximum.
If the function y = `(ax + b)/((x - 4)(x - 1))` has an extremum at P(2, –1), then the values of a and b are ______.
Let x and y be real numbers satisfying the equation x2 – 4x + y2 + 3 = 0. If the maximum and minimum values of x2 + y2 are a and b respectively. Then the numerical value of a – b is ______.
The sum of all the local minimum values of the twice differentiable function f : R `rightarrow` R defined by
f(x) = `x^3 - 3x^2 - (3f^('')(2))/2 x + f^('')(1)`
The minimum value of the function f(x) = xlogx is ______.
Find the maximum profit that a company can make, if the profit function is given by P(x) = 72 + 42x – x2, where x is the number of units and P is the profit in rupees.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) `= x sqrt(1 - x), 0 < x < 1`
Sumit has bought a closed cylindrical dustbin. The radius of the dustbin is ‘r' cm and height is 'h’ cm. It has a volume of 20π cm3.
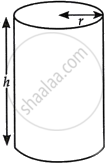
- Express ‘h’ in terms of ‘r’, using the given volume.
- Prove that the total surface area of the dustbin is `2πr^2 + (40π)/r`
- Sumit wants to paint the dustbin. The cost of painting the base and top of the dustbin is ₹ 2 per cm2 and the cost of painting the curved side is ₹ 25 per cm2. Find the total cost in terms of ‘r’, for painting the outer surface of the dustbin including the base and top.
- Calculate the minimum cost for painting the dustbin.
