Advertisements
Advertisements
प्रश्न
A metal box with a square base and vertical sides is to contain 1024 cm3. The material for the top and bottom costs Rs 5/cm2 and the material for the sides costs Rs 2.50/cm2. Find the least cost of the box.
उत्तर
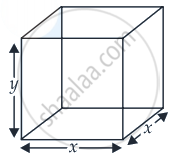
Let x be the side of the square base and y be the length of the vertical sides.
Area of the base and bottom = 2x2 cm2
∴ Cost of the material required = ₹ 5 × 2x2
= ₹ 10x2
Area of the 4 sides = 4xy cm2
∴ Cost of the material for the four sides
= ₹ 2.50 x 4xy
= ₹ 10xy
Total cost C = 10x2 + 10xy .....(i)
New volume of the box = x × x × y
⇒ 1024 = x2y
∴ y = `1024/x^2` ....(ii)
Putting the value of y in equation (i) we get
C = `10x^2 + 10x xx 1024/x^2`
⇒ C = `10x^2 + 10240/x`
Differentiating both sides w.r.t. x, we get
`"dC"/"dx" = 20x - 10240/x^2` ....(iii)
For local maxima and local minima `"dC"/"dx"` = 0
`20 - 102400/x^2` = 0
⇒ 20x3 – 10240 = 0
⇒ x3 = 512
⇒ x = 8 cm
Now from equation (ii)
y = `10240/(8)^2`
= `10240/64`
= 16 cm
∴ Cost of material used C = 10x2 + 10xy
= 10 × 8 × 8 + 10 × 8 × 16
= 640 + 1280
= 1920
Now differentiating equation (iii) we get
`("d"^2"C")/("dx"^2) = 20 + 20480/x^3`
Put x = 8
= `20 + 20480/(8)^3`
= `20 + 20480/512`
= 20 + 40 = 60 > 0 minima
Hence, the required cost is ₹ 1920 which is the minimum.
APPEARS IN
संबंधित प्रश्न
An open box is to be made out of a piece of a square card board of sides 18 cms by cutting off equal squares from the comers and turning up the sides. Find the maximum volume of the box.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) = sinx − cos x, 0 < x < 2π
Find the maximum profit that a company can make, if the profit function is given by p(x) = 41 − 72x − 18x2.
At what points in the interval [0, 2π], does the function sin 2x attain its maximum value?
It is given that at x = 1, the function x4− 62x2 + ax + 9 attains its maximum value, on the interval [0, 2]. Find the value of a.
Find two positive numbers x and y such that x + y = 60 and xy3 is maximum.
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is `(4r)/3.`
Show that the surface area of a closed cuboid with square base and given volume is minimum, when it is a cube.
Show that the cone of the greatest volume which can be inscribed in a given sphere has an altitude equal to \[ \frac{2}{3} \] of the diameter of the sphere.
Show that among rectangles of given area, the square has least perimeter.
Show that the height of a closed right circular cylinder of given volume and least surface area is equal to its diameter.
Solve the following : Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is `(2"R")/sqrt(3)`. Also, find the maximum volume.
If f(x) = 3x3 - 9x2 - 27x + 15, then the maximum value of f(x) is _______.
If R is the circum radius of Δ ABC, then A(Δ ABC) = ______.
If the sum of the lengths of the hypotenuse and a side of a right-angled triangle is given, show that the area of the triangle is maximum when the angle between them is `pi/3`
The function f(x) = 2x3 – 3x2 – 12x + 4, has ______.
Find the local minimum value of the function f(x) `= "sin"^4" x + cos"^4 "x", 0 < "x" < pi/2`
Find both the maximum and minimum values respectively of 3x4 - 8x3 + 12x2 - 48x + 1 on the interval [1, 4].
A ball is thrown upward at a speed of 28 meter per second. What is the speed of ball one second before reaching maximum height? (Given that g= 10 meter per second2)
For all real values of `x`, the minimum value of `(1 - x + x^2)/(1 + x + x^2)`
The minimum value of α for which the equation `4/sinx + 1/(1 - sinx)` = α has at least one solution in `(0, π/2)` is ______.
Let P(h, k) be a point on the curve y = x2 + 7x + 2, nearest to the line, y = 3x – 3. Then the equation of the normal to the curve at P is ______.
If p(x) be a polynomial of degree three that has a local maximum value 8 at x = 1 and a local minimum value 4 at x = 2; then p(0) is equal to ______.
If y = alog|x| + bx2 + x has its extremum values at x = –1 and x = 2, then ______.
The lateral edge of a regular rectangular pyramid is 'a' cm long. The lateral edge makes an angle a. with the plane of the base. The value of a for which the volume of the pyramid is greatest, is ______.
The maximum value of z = 6x + 8y subject to constraints 2x + y ≤ 30, x + 2y ≤ 24 and x ≥ 0, y ≥ 0 is ______.
Find the maximum and the minimum values of the function f(x) = x2ex.
A running track of 440 m is to be laid out enclosing a football field. The football field is in the shape of a rectangle with a semi-circle at each end. If the area of the rectangular portion is to be maximum,then find the length of its sides. Also calculate the area of the football field.
If x + y = 8, then the maximum value of x2y is ______.
