Advertisements
Advertisements
प्रश्न
A running track of 440 m is to be laid out enclosing a football field. The football field is in the shape of a rectangle with a semi-circle at each end. If the area of the rectangular portion is to be maximum,then find the length of its sides. Also calculate the area of the football field.
उत्तर
Let 2a and 2b be the sides of rectangular portion ABCD.
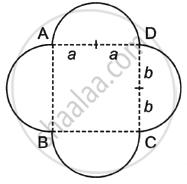
Given length of running track = 440 m
πa + πb + πa + πb = 440
`\implies` 2πa + 2πb = 440
`\implies 2 xx 22/7(a + b)` = 440
`\implies` a + b = 70 ...(1)
Area of Rectangle ABCD
A = (2a)(2b)
= 4ab
= 4a(70 – a)
= 4(70a – a2)
∴ `("dA")/("da")` = 4(70 – 2a)
For max/min `("dA")/("da")` = 0
4(70 – 2a) = 0
∴ a = 35
Also `("d"^2"A")/("da"^2)` = – 8a
= – 8 × 35 < 0
So, A is maximum when a = 35
By (1),
b = 70 – a
= 70 – 35
= 35
Hence sides of rectangular portion are
2a = 2 × 35 = 70 m
and 2b = 2 × 35 = 70 m
2nd part: Area of whole field
= `2 xx 1/2 π"a"^2 + 2 xx 1/2 π"b"^2 + 2"a" xx 2"b"`
= πa2 + πb2 + 4ab
= `22/7 xx (35)^2 + 22/7 xx (35)^2 + 4 xx 35 xx 35`
= 3850 + 3850 + 4900
= 12600
APPEARS IN
संबंधित प्रश्न
Find the approximate value of cos (89°, 30'). [Given is: 1° = 0.0175°C]
Find the maximum and minimum value, if any, of the following function given by f(x) = (2x − 1)2 + 3.
Find the maximum and minimum value, if any, of the following function given by g(x) = x3 + 1.
Find the maximum and minimum value, if any, of the function given by f(x) = |x + 2| − 1.
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
f (x) = sin x + cos x , x ∈ [0, π]
Find two positive numbers x and y such that x + y = 60 and xy3 is maximum.
The maximum value of `[x(x −1) +1]^(1/3)` , 0 ≤ x ≤ 1 is ______.
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is `(4r)/3.`
Show that a cylinder of a given volume, which is open at the top, has minimum total surface area when its height is equal to the radius of its base.
A ball is thrown in the air. Its height at any time t is given by h = 3 + 14t – 5t2. Find the maximum height it can reach.
The perimeter of a triangle is 10 cm. If one of the side is 4 cm. What are the other two sides of the triangle for its maximum area?
Divide the number 20 into two parts such that their product is maximum.
If z = ax + by; a, b > 0 subject to x ≤ 2, y ≤ 2, x + y ≥ 3, x ≥ 0, y ≥ 0 has minimum value at (2, 1) only, then ______.
Let f have second derivative at c such that f′(c) = 0 and f"(c) > 0, then c is a point of ______.
A metal box with a square base and vertical sides is to contain 1024 cm3. The material for the top and bottom costs Rs 5/cm2 and the material for the sides costs Rs 2.50/cm2. Find the least cost of the box.
If y `= "ax - b"/(("x" - 1)("x" - 4))` has a turning point P(2, -1), then find the value of a and b respectively.
Find the maximum profit that a company can make, if the profit function is given by P(x) = 41 + 24x – 18x2.
The combined resistance R of two resistors R1 and R2 (R1, R2 > 0) is given by `1/"R" = 1/"R"_1 + 1/"R"_2`. If R1 + R2 = C (a constant), then maximum resistance R is obtained if ____________.
For all real values of `x`, the minimum value of `(1 - x + x^2)/(1 + x + x^2)`
Read the following passage and answer the questions given below.
|
In an elliptical sport field the authority wants to design a rectangular soccer field with the maximum possible area. The sport field is given by the graph of `x^2/a^2 + y^2/b^2` = 1. |
- If the length and the breadth of the rectangular field be 2x and 2y respectively, then find the area function in terms of x.
- Find the critical point of the function.
- Use First derivative Test to find the length 2x and width 2y of the soccer field (in terms of a and b) that maximize its area.
OR
Use Second Derivative Test to find the length 2x and width 2y of the soccer field (in terms of a and b) that maximize its area.
A wire of length 22 m is to be cut into two pieces. One of the pieces is to be made into a square and the other into an equilateral triangle. Then, the length of the side of the equilateral triangle, so that the combined area of the square and the equilateral triangle is minimum, is ______.
If the point (1, 3) serves as the point of inflection of the curve y = ax3 + bx2 then the value of 'a ' and 'b' are ______.
The minimum value of 2sinx + 2cosx is ______.
A rectangle with one side lying along the x-axis is to be inscribed in the closed region of the xy plane bounded by the lines y = 0, y = 3x and y = 30 – 2x. The largest area of such a rectangle is ______.
A rod AB of length 16 cm. rests between the wall AD and a smooth peg, 1 cm from the wall and makes an angle θ with the horizontal. The value of θ for which the height of G, the midpoint of the rod above the peg is minimum, is ______.
Check whether the function f : R `rightarrow` R defined by f(x) = x3 + x, has any critical point/s or not ? If yes, then find the point/s.
A metal wire of 36 cm long is bent to form a rectangle. Find its dimensions when its area is maximum.
Complete the following activity to divide 84 into two parts such that the product of one part and square of the other is maximum.
Solution: Let one part be x. Then the other part is 84 - x
Letf (x) = x2 (84 - x) = 84x2 - x3
∴ f'(x) = `square`
and f''(x) = `square`
For extreme values, f'(x) = 0
∴ x = `square "or" square`
f(x) attains maximum at x = `square`
Hence, the two parts of 84 are 56 and 28.
Divide the number 100 into two parts so that the sum of their squares is minimum.

