Advertisements
Advertisements
Question
A running track of 440 m is to be laid out enclosing a football field. The football field is in the shape of a rectangle with a semi-circle at each end. If the area of the rectangular portion is to be maximum,then find the length of its sides. Also calculate the area of the football field.
Solution
Let 2a and 2b be the sides of rectangular portion ABCD.
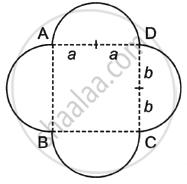
Given length of running track = 440 m
πa + πb + πa + πb = 440
`\implies` 2πa + 2πb = 440
`\implies 2 xx 22/7(a + b)` = 440
`\implies` a + b = 70 ...(1)
Area of Rectangle ABCD
A = (2a)(2b)
= 4ab
= 4a(70 – a)
= 4(70a – a2)
∴ `("dA")/("da")` = 4(70 – 2a)
For max/min `("dA")/("da")` = 0
4(70 – 2a) = 0
∴ a = 35
Also `("d"^2"A")/("da"^2)` = – 8a
= – 8 × 35 < 0
So, A is maximum when a = 35
By (1),
b = 70 – a
= 70 – 35
= 35
Hence sides of rectangular portion are
2a = 2 × 35 = 70 m
and 2b = 2 × 35 = 70 m
2nd part: Area of whole field
= `2 xx 1/2 π"a"^2 + 2 xx 1/2 π"b"^2 + 2"a" xx 2"b"`
= πa2 + πb2 + 4ab
= `22/7 xx (35)^2 + 22/7 xx (35)^2 + 4 xx 35 xx 35`
= 3850 + 3850 + 4900
= 12600
APPEARS IN
RELATED QUESTIONS
Examine the maxima and minima of the function f(x) = 2x3 - 21x2 + 36x - 20 . Also, find the maximum and minimum values of f(x).
If `f'(x)=k(cosx-sinx), f'(0)=3 " and " f(pi/2)=15`, find f(x).
An open box is to be made out of a piece of a square card board of sides 18 cms by cutting off equal squares from the comers and turning up the sides. Find the maximum volume of the box.
If the sum of lengths of hypotenuse and a side of a right angled triangle is given, show that area of triangle is maximum, when the angle between them is π/3.
Find the maximum and minimum value, if any, of the following function given by h(x) = sin(2x) + 5.
Find the point on the straight line 2x+3y = 6, which is closest to the origin.
Find the maximum and minimum of the following functions : y = 5x3 + 2x2 – 3x.
Find the largest size of a rectangle that can be inscribed in a semicircle of radius 1 unit, so that two vertices lie on the diameter.
Solve the following : An open box with a square base is to be made out of given quantity of sheet of area a2. Show that the maximum volume of the box is `a^3/(6sqrt(3)`.
Determine the maximum and minimum value of the following function.
f(x) = `x^2 + 16/x`
Divide the number 20 into two parts such that their product is maximum.
State whether the following statement is True or False:
An absolute maximum must occur at a critical point or at an end point.
Max value of z equals 3x + 2y subject to x + y ≤ 3, x ≤ 2, -2x + y ≤ 1, x ≥ 0, y ≥ 0 is ______
If f(x) = `x + 1/x, x ne 0`, then local maximum and x minimum values of function f are respectively.
The function f(x) = 2x3 – 3x2 – 12x + 4, has ______.
A ball is thrown upward at a speed of 28 meter per second. What is the speed of ball one second before reaching maximum height? (Given that g= 10 meter per second2)
The minimum value of α for which the equation `4/sinx + 1/(1 - sinx)` = α has at least one solution in `(0, π/2)` is ______.
The range of a ∈ R for which the function f(x) = `(4a - 3)(x + log_e5) + 2(a - 7)cot(x/2)sin^2(x/2), x ≠ 2nπ, n∈N` has critical points, is ______.
A cone of maximum volume is inscribed in a given sphere. Then the ratio of the height of the cone to the diameter of the sphere is ______.
Let f(x) = |(x – 1)(x2 – 2x – 3)| + x – 3, x ∈ R. If m and M are respectively the number of points of local minimum and local maximum of f in the interval (0, 4), then m + M is equal to ______.
The maximum distance from origin of a point on the curve x = `a sin t - b sin((at)/b)`, y = `a cos t - b cos((at)/b)`, both a, b > 0 is ______.
A rectangle with one side lying along the x-axis is to be inscribed in the closed region of the xy plane bounded by the lines y = 0, y = 3x and y = 30 – 2x. The largest area of such a rectangle is ______.
The volume of the greatest cylinder which can be inscribed in a cone of height 30 cm and semi-vertical angle 30° is ______.
The maximum value of f(x) = `logx/x (x ≠ 0, x ≠ 1)` is ______.
The point in the interval [0, 2π], where f(x) = ex sin x has maximum slope, is ______.
Sum of two numbers is 5. If the sum of the cubes of these numbers is least, then find the sum of the squares of these numbers.
The rectangle has area of 50 cm2. Complete the following activity to find its dimensions for least perimeter.
Solution: Let x cm and y cm be the length and breadth of a rectangle.
Then its area is xy = 50
∴ `y =50/x`
Perimeter of rectangle `=2(x+y)=2(x+50/x)`
Let f(x) `=2(x+50/x)`
Then f'(x) = `square` and f''(x) = `square`
Now,f'(x) = 0, if x = `square`
But x is not negative.
∴ `x = root(5)(2) "and" f^('')(root(5)(2))=square>0`
∴ by the second derivative test f is minimum at x = `root(5)(2)`
When x = `root(5)(2),y=50/root(5)(2)=root(5)(2)`
∴ `x=root(5)(2) "cm" , y = root(5)(2) "cm"`
Hence, rectangle is a square of side `root(5)(2) "cm"`
