Advertisements
Advertisements
प्रश्न
A running track of 440 m is to be laid out enclosing a football field. The football field is in the shape of a rectangle with a semi-circle at each end. If the area of the rectangular portion is to be maximum,then find the length of its sides. Also calculate the area of the football field.
उत्तर
Let 2a and 2b be the sides of rectangular portion ABCD.
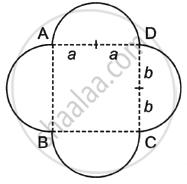
Given length of running track = 440 m
πa + πb + πa + πb = 440
`\implies` 2πa + 2πb = 440
`\implies 2 xx 22/7(a + b)` = 440
`\implies` a + b = 70 ...(1)
Area of Rectangle ABCD
A = (2a)(2b)
= 4ab
= 4a(70 – a)
= 4(70a – a2)
∴ `("dA")/("da")` = 4(70 – 2a)
For max/min `("dA")/("da")` = 0
4(70 – 2a) = 0
∴ a = 35
Also `("d"^2"A")/("da"^2)` = – 8a
= – 8 × 35 < 0
So, A is maximum when a = 35
By (1),
b = 70 – a
= 70 – 35
= 35
Hence sides of rectangular portion are
2a = 2 × 35 = 70 m
and 2b = 2 × 35 = 70 m
2nd part: Area of whole field
= `2 xx 1/2 π"a"^2 + 2 xx 1/2 π"b"^2 + 2"a" xx 2"b"`
= πa2 + πb2 + 4ab
= `22/7 xx (35)^2 + 22/7 xx (35)^2 + 4 xx 35 xx 35`
= 3850 + 3850 + 4900
= 12600
APPEARS IN
संबंधित प्रश्न
A telephone company in a town has 5000 subscribers on its list and collects fixed rent charges of Rs.3,000 per year from each subscriber. The company proposes to increase annual rent and it is believed that for every increase of one rupee in the rent, one subscriber will be discontinued. Find what increased annual rent will bring the maximum annual income to the company.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`g(x) = 1/(x^2 + 2)`
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
`f(x) = 4x - 1/x x^2, x in [-2 ,9/2]`
Find the maximum profit that a company can make, if the profit function is given by p(x) = 41 − 72x − 18x2.
Find both the maximum value and the minimum value of 3x4 − 8x3 + 12x2 − 48x + 25 on the interval [0, 3].
Of all the closed cylindrical cans (right circular), of a given volume of 100 cubic centimetres, find the dimensions of the can which has the minimum surface area?
Find the points at which the function f given by f (x) = (x – 2)4 (x + 1)3 has
- local maxima
- local minima
- point of inflexion
An open tank with a square base and vertical sides is to be constructed from a metal sheet so as to hold a given quantity of water. Show that the cost of material will be least when the depth of the tank is half of its width. If the cost is to be borne by nearby settled lower-income families, for whom water will be provided, what kind of value is hidden in this question?
A given quantity of metal is to be cast into a half cylinder with a rectangular base and semicircular ends. Show that in order that the total surface area may be minimum the ratio of the length of the cylinder to the diameter of its semi-circular ends is \[\pi : (\pi + 2)\].
Show that a cylinder of a given volume, which is open at the top, has minimum total surface area when its height is equal to the radius of its base.
Find the point on the straight line 2x+3y = 6, which is closest to the origin.
Find the maximum and minimum of the following functions : f(x) = x log x
Divide the number 30 into two parts such that their product is maximum.
Find the largest size of a rectangle that can be inscribed in a semicircle of radius 1 unit, so that two vertices lie on the diameter.
Determine the maximum and minimum value of the following function.
f(x) = x log x
Determine the maximum and minimum value of the following function.
f(x) = `x^2 + 16/x`
A metal wire of 36cm long is bent to form a rectangle. Find it's dimensions when it's area is maximum.
Max value of z equals 3x + 2y subject to x + y ≤ 3, x ≤ 2, -2x + y ≤ 1, x ≥ 0, y ≥ 0 is ______
The function y = 1 + sin x is maximum, when x = ______
If x is real, the minimum value of x2 – 8x + 17 is ______.
The function f(x) = 2x3 – 3x2 – 12x + 4, has ______.
Find the points of local maxima and local minima respectively for the function f(x) = sin 2x - x, where `-pi/2 le "x" le pi/2`
A ball is thrown upward at a speed of 28 meter per second. What is the speed of ball one second before reaching maximum height? (Given that g= 10 meter per second2)
A wire of length 36 m is cut into two pieces, one of the pieces is bent to form a square and the other is bent to form a circle. If the sum of the areas of the two figures is minimum, and the circumference of the circle is k (meter), then `(4/π + 1)`k is equal to ______.
The volume of the greatest cylinder which can be inscribed in a cone of height 30 cm and semi-vertical angle 30° is ______.
Read the following passage:
Engine displacement is the measure of the cylinder volume swept by all the pistons of a piston engine. The piston moves inside the cylinder bore.
|
Based on the above information, answer the following questions:
- If the radius of cylinder is r cm and height is h cm, then write the volume V of cylinder in terms of radius r. (1)
- Find `(dV)/(dr)`. (1)
- (a) Find the radius of cylinder when its volume is maximum. (2)
OR
(b) For maximum volume, h > r. State true or false and justify. (2)
Sum of two numbers is 5. If the sum of the cubes of these numbers is least, then find the sum of the squares of these numbers.
Complete the following activity to divide 84 into two parts such that the product of one part and square of the other is maximum.
Solution: Let one part be x. Then the other part is 84 - x
Letf (x) = x2 (84 - x) = 84x2 - x3
∴ f'(x) = `square`
and f''(x) = `square`
For extreme values, f'(x) = 0
∴ x = `square "or" square`
f(x) attains maximum at x = `square`
Hence, the two parts of 84 are 56 and 28.
If Mr. Rane order x chairs at the price p = (2x2 - 12x - 192) per chair. How many chairs should he order so that the cost of deal is minimum?
Solution: Let Mr. Rane order x chairs.
Then the total price of x chairs = p·x = (2x2 - 12x- 192)x
= 2x3 - 12x2 - 192x
Let f(x) = 2x3 - 12x2 - 192x
∴ f'(x) = `square` and f''(x) = `square`
f'(x ) = 0 gives x = `square` and f''(8) = `square` > 0
∴ f is minimum when x = 8
Hence, Mr. Rane should order 8 chairs for minimum cost of deal.
A box with a square base is to have an open top. The surface area of box is 147 sq. cm. What should be its dimensions in order that the volume is largest?



