Advertisements
Advertisements
प्रश्न
Show that the cone of the greatest volume which can be inscribed in a given sphere has an altitude equal to \[ \frac{2}{3} \] of the diameter of the sphere.
A cone of maximum volume is inscribed in a given sphere. Then prove that ratio of the height of the cone to the diameter of the sphere is equal to `2/3`.
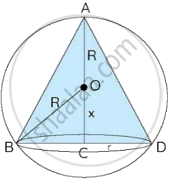
उत्तर
\[\text{Let h, r and R be the height, radius of base of the cone and radius of the sphere, respectively. Then},\]
\[h = R + \sqrt{R^2 - r^2}\]
\[\Rightarrow\left( h - R \right)^2 = R^2 - r^2\]
\[\Rightarrow h^2 + R^2 - 2hr = R^2 - r^2\]
\[\Rightarrow r^2 = 2hR - h^2 ........\left(1 \right)\]
\[\text{Volume of cone} = \frac{1}{3}\pi r^2 h\]
\[\Rightarrow V = \frac{1}{3}\pi h\left(2hR - h^2 \right) .............\left[\text {From equation}\left( 1 \right) \right]\]
\[\Rightarrow V = \frac{1}{3}\pi\left(2 h^2 R - h^3 \right)\]
\[\Rightarrow \frac{dV}{dh} = \frac{\pi}{3}\left(4hR - 3 h^2 \right)\]
\[\text{For maximum or minimum values of V, we must have}\]
\[\frac{dV}{dh} = 0\]
\[\Rightarrow \frac{\pi}{3}\left( 4hR - 3 h^2\right) = 0\]
\[\Rightarrow 4hR = 3 h^2 \]
\[\Rightarrow h = \frac{4R}{3}\]
\[\text{Substituting the value of y in equation} \left(1 \right),\text {we get}\]
\[x^2 = 4\left( r^2 - \left(\frac{r}{\sqrt{2}} \right)^2\right)\]
\[\Rightarrow x^2 = 4\left(r^2 - \frac{r^2}{2}\right)\]
\[\Rightarrow x^2 = 4\left(\frac{r^2}{2}\right)\]
\[\Rightarrow x^2 = 2 r^2\]
\[\Rightarrow x = r\sqrt{2}\]
\[\text{Now,}\]
\[\frac{d^2 V}{d h^2} = \frac{\pi}{3}\left(4R - 6h \right)\]
\[\Rightarrow \frac{d^2 V}{d h^2} = \frac{\pi}{3}\left( 4R - 6 \times \frac{4R}{3} \right)\]
\[\Rightarrow \frac{d^2 V}{d h^2} = \frac{- 4\pi R}{3} < 0\]
\[\text{So, the volume is maximum when h} = \frac{4R}{3}.\]
\[\Rightarrow h = \frac{2}{3}\left( \text {Diameter of sphere}\right)\]
\[\text{Hence proved}.\]
संबंधित प्रश्न
Show that the height of the cylinder of maximum volume, that can be inscribed in a sphere of radius R is `(2R)/sqrt3.` Also, find the maximum volume.
An open box is to be made out of a piece of a square card board of sides 18 cms by cutting off equal squares from the comers and turning up the sides. Find the maximum volume of the box.
Find the maximum and minimum value, if any, of the following function given by g(x) = x3 + 1.
Find the maximum and minimum value, if any, of the following function given by g(x) = − |x + 1| + 3.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
g(x) = x3 − 3x
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) = sinx − cos x, 0 < x < 2π
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`g(x) = 1/(x^2 + 2)`
Find the local maxima and local minima, if any, of the following functions. Find also the local maximum and the local minimum values, as the case may be:
`f(x) = xsqrt(1-x), x > 0`
Prove that the following function do not have maxima or minima:
f(x) = ex
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
`f(x) =x^3, x in [-2,2]`
At what points in the interval [0, 2π], does the function sin 2x attain its maximum value?
Show that the right circular cylinder of given surface and maximum volume is such that is heights is equal to the diameter of the base.
Of all the closed cylindrical cans (right circular), of a given volume of 100 cubic centimetres, find the dimensions of the can which has the minimum surface area?
A wire of length 28 m is to be cut into two pieces. One of the pieces is to be made into a square and the other into a circle. What should be the length of the two pieces so that the combined area of the square and the circle is minimum?
The point on the curve x2 = 2y which is nearest to the point (0, 5) is ______.
The maximum value of `[x(x −1) +1]^(1/3)` , 0 ≤ x ≤ 1 is ______.
Find the absolute maximum and minimum values of the function f given by f (x) = cos2 x + sin x, x ∈ [0, π].
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is `(4r)/3.`
A metal box with a square base and vertical sides is to contain 1024 cm3. The material for the top and bottom costs Rs 5 per cm2 and the material for the sides costs Rs 2.50 per cm2. Find the least cost of the box
Prove that the semi-vertical angle of the right circular cone of given volume and least curved surface is \[\cot^{- 1} \left( \sqrt{2} \right)\] .
Find the maximum and minimum of the following functions : f(x) = x3 – 9x2 + 24x
Find the maximum and minimum of the following functions : f(x) = `x^2 + (16)/x^2`
Divide the number 20 into two parts such that sum of their squares is minimum.
An open cylindrical tank whose base is a circle is to be constructed of metal sheet so as to contain a volume of `pia^3`cu cm of water. Find the dimensions so that the quantity of the metal sheet required is minimum.
Choose the correct option from the given alternatives :
If f(x) = `(x^2 - 1)/(x^2 + 1)`, for every real x, then the minimum value of f is ______.
Solve the following : Show that a closed right circular cylinder of given surface area has maximum volume if its height equals the diameter of its base.
Solve the following:
A rectangular sheet of paper of fixed perimeter with the sides having their lengths in the ratio 8 : 15 converted into an open rectangular box by folding after removing the squares of equal area from all corners. If the total area of the removed squares is 100, the resulting box has maximum volume. Find the lengths of the rectangular sheet of paper.
Determine the maximum and minimum value of the following function.
f(x) = x log x
State whether the following statement is True or False:
An absolute maximum must occur at a critical point or at an end point.
If x + y = 3 show that the maximum value of x2y is 4.
A rod of 108 m long is bent to form a rectangle. Find it’s dimensions when it’s area is maximum.
By completing the following activity, examine the function f(x) = x3 – 9x2 + 24x for maxima and minima
Solution: f(x) = x3 – 9x2 + 24x
∴ f'(x) = `square`
∴ f''(x) = `square`
For extreme values, f'(x) = 0, we get
x = `square` or `square`
∴ f''`(square)` = – 6 < 0
∴ f(x) is maximum at x = 2.
∴ Maximum value = `square`
∴ f''`(square)` = 6 > 0
∴ f(x) is maximum at x = 4.
∴ Minimum value = `square`
The maximum volume of a right circular cylinder if the sum of its radius and height is 6 m is ______.
If f(x) = 3x3 - 9x2 - 27x + 15, then the maximum value of f(x) is _______.
If R is the circum radius of Δ ABC, then A(Δ ABC) = ______.
If the sum of the lengths of the hypotenuse and a side of a right-angled triangle is given, show that the area of the triangle is maximum when the angle between them is `pi/3`
The maximum value of sin x . cos x is ______.
Find the local minimum value of the function f(x) `= "sin"^4" x + cos"^4 "x", 0 < "x" < pi/2`
If y `= "ax - b"/(("x" - 1)("x" - 4))` has a turning point P(2, -1), then find the value of a and b respectively.
Find the height of the cylinder of maximum volume that can be inscribed in a sphere of radius a.
Find the area of the largest isosceles triangle having a perimeter of 18 meters.
The distance of that point on y = x4 + 3x2 + 2x which is nearest to the line y = 2x - 1 is ____________.
The function `f(x) = x^3 - 6x^2 + 9x + 25` has
For all real values of `x`, the minimum value of `(1 - x + x^2)/(1 + x + x^2)`
Read the following passage and answer the questions given below.
|
|
- Is the function differentiable in the interval (0, 12)? Justify your answer.
- If 6 is the critical point of the function, then find the value of the constant m.
- Find the intervals in which the function is strictly increasing/strictly decreasing.
OR
Find the points of local maximum/local minimum, if any, in the interval (0, 12) as well as the points of absolute maximum/absolute minimum in the interval [0, 12]. Also, find the corresponding local maximum/local minimum and the absolute ‘maximum/absolute minimum values of the function.
A function f(x) is maximum at x = a when f'(a) > 0.
A wire of length 22 m is to be cut into two pieces. One of the pieces is to be made into a square and the other into an equilateral triangle. Then, the length of the side of the equilateral triangle, so that the combined area of the square and the equilateral triangle is minimum, is ______.
Let f: R → R be a function defined by f(x) = (x – 3)n1(x – 5)n2, n1, n2 ∈ N. Then, which of the following is NOT true?
A wire of length 36 m is cut into two pieces, one of the pieces is bent to form a square and the other is bent to form a circle. If the sum of the areas of the two figures is minimum, and the circumference of the circle is k (meter), then `(4/π + 1)`k is equal to ______.
Let P(h, k) be a point on the curve y = x2 + 7x + 2, nearest to the line, y = 3x – 3. Then the equation of the normal to the curve at P is ______.
If S1 and S2 are respectively the sets of local minimum and local maximum points of the function. f(x) = 9x4 + 12x3 – 36x2 + 25, x ∈ R, then ______.
If the function y = `(ax + b)/((x - 4)(x - 1))` has an extremum at P(2, –1), then the values of a and b are ______.
The greatest value of the function f(x) = `tan^-1x - 1/2logx` in `[1/sqrt(3), sqrt(3)]` is ______.
The minimum value of 2sinx + 2cosx is ______.
The maximum value of z = 6x + 8y subject to constraints 2x + y ≤ 30, x + 2y ≤ 24 and x ≥ 0, y ≥ 0 is ______.
The minimum value of the function f(x) = xlogx is ______.
Complete the following activity to divide 84 into two parts such that the product of one part and square of the other is maximum.
Solution: Let one part be x. Then the other part is 84 - x
Letf (x) = x2 (84 - x) = 84x2 - x3
∴ f'(x) = `square`
and f''(x) = `square`
For extreme values, f'(x) = 0
∴ x = `square "or" square`
f(x) attains maximum at x = `square`
Hence, the two parts of 84 are 56 and 28.
A running track of 440 m is to be laid out enclosing a football field. The football field is in the shape of a rectangle with a semi-circle at each end. If the area of the rectangular portion is to be maximum,then find the length of its sides. Also calculate the area of the football field.
A box with a square base is to have an open top. The surface area of box is 147 sq. cm. What should be its dimensions in order that the volume is largest?
Determine the minimum value of the function.
f(x) = 2x3 – 21x2 + 36x – 20

