Advertisements
Advertisements
प्रश्न
Solve the following : Show that a closed right circular cylinder of given surface area has maximum volume if its height equals the diameter of its base.
उत्तर
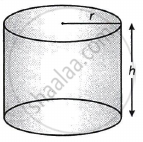
Let r be the radius of the base, h be the height and V be the volume of the closed right circular cylinder, whose surface area is a2 sq. units (which is given).
∴ 2πrh + 2πr2 = a2
∴ 2πr(h + r) = a2
∴ h = `a^2/(2πr) - r` ...(1)
Now, V = πr2h
= `πr^2(a^2/(2πr) - r)` ...[By (1)]
= `(1)/(2)a^2r - πr^3`
∴ `(dV)/(dr) = d/(dr)(1/2a^2r - πr^3)`
= `(1)/(2)a^2 xx 1 - π xx 3r^2`
= `a^2/(2) - 3πr^2`
and
`(d^2V)/(dr^2) = d/(dr)(a^2/2 - 3πr^2)`
= 0 – 3π × 2r
= – 6πr
For maximum volume,
`(dV)/(dr)` = 0
∴ `a^2/(2) - 3πr^2` = 0
∴ 3πr2 = `a^2/(2)`
∴ r2 = `a^2/(6π)`
∴ r = `a/sqrt(6π)` ...[∵ r > 0]
and
`((d^2V)/(dx^2))_(at r = a/sqrt(6π)`
= `- 6π(a/sqrt(6π)) < 0`
∴ V is maximum when r = `a/sqrt(6π)`
When r = `a/sqrt(6π)`, then from (1),
h = `a^2/(2π xx a/sqrt(6π)) - a/sqrt(6π)`
= `sqrt(6πa)/(2π) - a/sqrt(6π)`
= `(6πa - 2πa)/(2πsqrt(6π)`
= `(4πa)/(2πsqrt(6π)`
= `(2a)/sqrt(6π)`
∴ h = 2r
Hence, the volume of the cylinder is maximum if its height is equal to the diameter of the base.
APPEARS IN
संबंधित प्रश्न
Find the approximate value of cos (89°, 30'). [Given is: 1° = 0.0175°C]
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is `(4r)/3`. Also find maximum volume in terms of volume of the sphere
Find the maximum and minimum value, if any, of the function given by f(x) = |x + 2| − 1.
Find the maximum and minimum value, if any, of the following function given by h(x) = sin(2x) + 5.
Find the maximum and minimum value, if any, of the following function given by h(x) = x + 1, x ∈ (−1, 1)
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
g(x) = x3 − 3x
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`h(x) = sinx + cosx, 0 < x < pi/2`
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) = x3 − 6x2 + 9x + 15
Find the local maxima and local minima, if any, of the following functions. Find also the local maximum and the local minimum values, as the case may be:
`f(x) = xsqrt(1-x), x > 0`
Prove that the following function do not have maxima or minima:
f(x) = ex
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
`f(x) = 4x - 1/x x^2, x in [-2 ,9/2]`
Find the maximum profit that a company can make, if the profit function is given by p(x) = 41 − 72x − 18x2.
Find the maximum value of 2x3 − 24x + 107 in the interval [1, 3]. Find the maximum value of the same function in [−3, −1].
It is given that at x = 1, the function x4− 62x2 + ax + 9 attains its maximum value, on the interval [0, 2]. Find the value of a.
Find two positive numbers whose sum is 16 and the sum of whose cubes is minimum.
Show that of all the rectangles inscribed in a given fixed circle, the square has the maximum area.
Show that the right circular cylinder of given surface and maximum volume is such that is heights is equal to the diameter of the base.
The point on the curve x2 = 2y which is nearest to the point (0, 5) is ______.
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is `(4r)/3.`
Prove that the semi-vertical angle of the right circular cone of given volume and least curved surface is \[\cot^{- 1} \left( \sqrt{2} \right)\] .
The volume of a closed rectangular metal box with a square base is 4096 cm3. The cost of polishing the outer surface of the box is Rs. 4 per cm2. Find the dimensions of the box for the minimum cost of polishing it.
Find the maximum and minimum of the following functions : f(x) = x3 – 9x2 + 24x
Find the maximum and minimum of the following functions : f(x) = `x^2 + (16)/x^2`
Find the maximum and minimum of the following functions : f(x) = x log x
Divide the number 30 into two parts such that their product is maximum.
A wire of length 36 metres is bent in the form of a rectangle. Find its dimensions if the area of the rectangle is maximum.
The perimeter of a triangle is 10 cm. If one of the side is 4 cm. What are the other two sides of the triangle for its maximum area?
A box with a square base is to have an open top. The surface area of the box is 192 sq cm. What should be its dimensions in order that the volume is largest?
Show that among rectangles of given area, the square has least perimeter.
Choose the correct option from the given alternatives :
If f(x) = `(x^2 - 1)/(x^2 + 1)`, for every real x, then the minimum value of f is ______.
Solve the following : An open box with a square base is to be made out of given quantity of sheet of area a2. Show that the maximum volume of the box is `a^3/(6sqrt(3)`.
Solve the following : A window is in the form of a rectangle surmounted by a semicircle. If the perimeter be 30 m, find the dimensions so that the greatest possible amount of light may be admitted.
Solve the following : Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is `(2"R")/sqrt(3)`. Also, find the maximum volume.
Solve the following:
Find the maximum and minimum values of the function f(x) = cos2x + sinx.
Determine the maximum and minimum value of the following function.
f(x) = x log x
The total cost of producing x units is ₹ (x2 + 60x + 50) and the price is ₹ (180 − x) per unit. For what units is the profit maximum?
If f(x) = x.log.x then its maximum value is ______.
Examine the function for maxima and minima f(x) = x3 - 9x2 + 24x
The function f(x) = x log x is minimum at x = ______.
A wire of length 120 cm is bent in the form of a rectangle. Find its dimensions if the area of the rectangle is maximum
A rod of 108 m long is bent to form a rectangle. Find it’s dimensions when it’s area is maximum.
By completing the following activity, examine the function f(x) = x3 – 9x2 + 24x for maxima and minima
Solution: f(x) = x3 – 9x2 + 24x
∴ f'(x) = `square`
∴ f''(x) = `square`
For extreme values, f'(x) = 0, we get
x = `square` or `square`
∴ f''`(square)` = – 6 < 0
∴ f(x) is maximum at x = 2.
∴ Maximum value = `square`
∴ f''`(square)` = 6 > 0
∴ f(x) is maximum at x = 4.
∴ Minimum value = `square`
The maximum volume of a right circular cylinder if the sum of its radius and height is 6 m is ______.
If R is the circum radius of Δ ABC, then A(Δ ABC) = ______.
If z = ax + by; a, b > 0 subject to x ≤ 2, y ≤ 2, x + y ≥ 3, x ≥ 0, y ≥ 0 has minimum value at (2, 1) only, then ______.
The maximum value of function x3 - 15x2 + 72x + 19 in the interval [1, 10] is ______.
Let f have second derivative at c such that f′(c) = 0 and f"(c) > 0, then c is a point of ______.
Find the points of local maxima, local minima and the points of inflection of the function f(x) = x5 – 5x4 + 5x3 – 1. Also find the corresponding local maximum and local minimum values.
The sum of the surface areas of a rectangular parallelopiped with sides x, 2x and `x/3` and a sphere is given to be constant. Prove that the sum of their volumes is minimum, if x is equal to three times the radius of the sphere. Also find the minimum value of the sum of their volumes.
The area of a right-angled triangle of the given hypotenuse is maximum when the triangle is ____________.
The combined resistance R of two resistors R1 and R2 (R1, R2 > 0) is given by `1/"R" = 1/"R"_1 + 1/"R"_2`. If R1 + R2 = C (a constant), then maximum resistance R is obtained if ____________.
A ball is thrown upward at a speed of 28 meter per second. What is the speed of ball one second before reaching maximum height? (Given that g= 10 meter per second2)
For all real values of `x`, the minimum value of `(1 - x + x^2)/(1 + x + x^2)`
The maximum value of the function f(x) = `logx/x` is ______.
Divide 20 into two ports, so that their product is maximum.
Read the following passage and answer the questions given below.
|
|
- Is the function differentiable in the interval (0, 12)? Justify your answer.
- If 6 is the critical point of the function, then find the value of the constant m.
- Find the intervals in which the function is strictly increasing/strictly decreasing.
OR
Find the points of local maximum/local minimum, if any, in the interval (0, 12) as well as the points of absolute maximum/absolute minimum in the interval [0, 12]. Also, find the corresponding local maximum/local minimum and the absolute ‘maximum/absolute minimum values of the function.
Read the following passage and answer the questions given below.
|
In an elliptical sport field the authority wants to design a rectangular soccer field with the maximum possible area. The sport field is given by the graph of `x^2/a^2 + y^2/b^2` = 1. |
- If the length and the breadth of the rectangular field be 2x and 2y respectively, then find the area function in terms of x.
- Find the critical point of the function.
- Use First derivative Test to find the length 2x and width 2y of the soccer field (in terms of a and b) that maximize its area.
OR
Use Second Derivative Test to find the length 2x and width 2y of the soccer field (in terms of a and b) that maximize its area.
A wire of length 22 m is to be cut into two pieces. One of the pieces is to be made into a square and the other into an equilateral triangle. Then, the length of the side of the equilateral triangle, so that the combined area of the square and the equilateral triangle is minimum, is ______.
Let A = [aij] be a 3 × 3 matrix, where
aij = `{{:(1, "," if "i" = "j"),(-x, "," if |"i" - "j"| = 1),(2x + 1, "," "otherwise"):}`
Let a function f: R→R be defined as f(x) = det(A). Then the sum of maximum and minimum values of f on R is equal to ______.
If the point (1, 3) serves as the point of inflection of the curve y = ax3 + bx2 then the value of 'a ' and 'b' are ______.
The function g(x) = `(f(x))/x`, x ≠ 0 has an extreme value when ______.
The sum of all the local minimum values of the twice differentiable function f : R `rightarrow` R defined by
f(x) = `x^3 - 3x^2 - (3f^('')(2))/2 x + f^('')(1)`
The volume of the greatest cylinder which can be inscribed in a cone of height 30 cm and semi-vertical angle 30° is ______.
Check whether the function f : R `rightarrow` R defined by f(x) = x3 + x, has any critical point/s or not ? If yes, then find the point/s.
The rectangle has area of 50 cm2. Complete the following activity to find its dimensions for least perimeter.
Solution: Let x cm and y cm be the length and breadth of a rectangle.
Then its area is xy = 50
∴ `y =50/x`
Perimeter of rectangle `=2(x+y)=2(x+50/x)`
Let f(x) `=2(x+50/x)`
Then f'(x) = `square` and f''(x) = `square`
Now,f'(x) = 0, if x = `square`
But x is not negative.
∴ `x = root(5)(2) "and" f^('')(root(5)(2))=square>0`
∴ by the second derivative test f is minimum at x = `root(5)(2)`
When x = `root(5)(2),y=50/root(5)(2)=root(5)(2)`
∴ `x=root(5)(2) "cm" , y = root(5)(2) "cm"`
Hence, rectangle is a square of side `root(5)(2) "cm"`
Find the maximum and the minimum values of the function f(x) = x2ex.
If x + y = 8, then the maximum value of x2y is ______.
Determine the minimum value of the function.
f(x) = 2x3 – 21x2 + 36x – 20
Mrs. Roy designs a window in her son’s study room so that the room gets maximum sunlight. She designs the window in the shape of a rectangle surmounted by an equilateral triangle. If the perimeter of the window is 12 m, find the dimensions of the window that will admit maximum sunlight into the room.