Advertisements
Advertisements
प्रश्न
Find the area of a sector of a circle with radius 6 cm if angle of the sector is 60° [Use `pi = 22/7`]
उत्तर
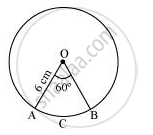
Let OACB be a sector of the circle making 60° angle at centre O of the circle.
Area of sector of angle θ = `theta/360^@xxpir^2`
Area of sector OACB = `60^@/360^@xx22/7xx(6)^2`
`=1/6xx22/7xx6xx6= 132/7 cm^2`
Therefore, the area of the sector of the circle making 60° at the centre of the circle is 132/7 cm2
APPEARS IN
संबंधित प्रश्न
Find the area of sector whose arc length and radius are 10 cm and 5 cm respectively
In the following figure, if m(are DXE) = 90° and m(are AYC) = 30°. Find ∠DBE.

In Fig. 3, AP and BP are tangents to a circle with centre O, such that AP = 5 cm and ∠APB = 60°. Find the length of chord AB.
The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes. [Use `pi = 22/7`]
The perimeter of a sector of circle of radius 5.7m is 27.2 m. Find the area of sector.
A chord 10 cm long is drawn in a circle whose radius is 5√2 cm. Find the area of both
segments
A chord AB of circle, of radius 14cm makes an angle of 60° at the centre. Find the area of minor segment of circle.
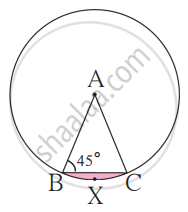
In the given figure, A is the center of the circle. ∠ABC = 45° and AC = 7√2 cm. Find the area of segment BXC.
In the given figure, if A is the centre of the circle. \[\angle\] PAR = 30°, AP = 7.5, find the area of the segment PQR. (\[\pi\] = 3.14)

In the given figure, APB and CQD are semicircles of diameter 7 cm each, while ARC an BSD are semicircles of diameter 14 cm each. Find the
- perimeter
- area of the shaded region.
The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
Find the area of the sector of a circle of radius 8 cm and arc with length 15 cm.
Find the area of the shaded region where ABC is a quadrant of radius 5cm and a semicircle is drawn with BC as diameter.

The number of revolutions made by a circular wheel of radius 0.7m in rolling a distance of 176m is ______.
The circumference of a circle is 100 cm. The side of a square inscribed in the circle is ______.
The areas of two sectors of two different circles with equal corresponding arc lengths are equal. Is this statement true? Why?
Is it true to say that area of a segment of a circle is less than the area of its corresponding sector? Why?
The central angles of two sectors of circles of radii 7 cm and 21 cm are respectively 120° and 40°. Find the areas of the two sectors as well as the lengths of the corresponding arcs. What do you observe?
The length of the minute hand of a clock is 6 cm. Find the area swept by it when it moves from 7:05 p.m. to 7:40 p.m.
The area of the sector of a circle of radius 12 cm is 60π cm2. The central angle of this sector is ______.
