Advertisements
Advertisements
प्रश्न
A chord AB of circle, of radius 14cm makes an angle of 60° at the centre. Find the area of minor segment of circle.
उत्तर
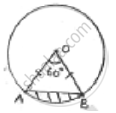
Given radius (r) = 14cm = OA = OB
𝜃 = angle at centre = 60°
In ΔAOB, ∠A = ∠B [angles opposite to equal sides OA and OB] = x
By angle sum property ∠A + ∠B + ∠O = 180°
x + x + 60° = 180° ⇒ 2x = 120° ⇒ x = 60°
All angles are 60°, OAB is equilateral OA = OB = AB
Area of segment = area of sector – area Δle OAB
=`theta/360^@× pir^2 − sqrt(3)/4× (−AB)^2`
=`60/360×22/7× 14 × 14 − sqrt(3)/4× 14 × 14`
=`308/3− 49sqrt(3)`
`= (308−147sqrt(3))/3cm^2`
APPEARS IN
संबंधित प्रश्न
A horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope (see the given figure). Find
- The area of that part of the field in which the horse can graze.
- The increase in the grazing area of the rope were 10 m long instead of 5 m. [Use π = 3.14]

A round table cover has six equal designs, as shown in figure. If the radius of the cover is 28 cm, find the cost of making the designs at the rate of Rs.0.35 per cm2. [Use `sqrt3 = 1.7`]
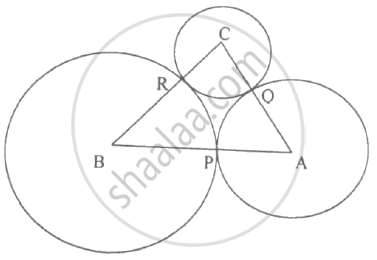
ABC is a triangle with AB = 10 cm, BC = 8 cm and AC = 6 cm (not drawn to scale). Three circles are drawn touching each other with the vertices as their centres. Find the radii of the three circles.
A chord of circle of radius 14cm makes a right angle at the centre. Find the areas of minor and major segments of the circle.
A chord of a circle subtends an angle 𝜃 at the centre of circle. The area of the minor segment cut off by the chord is one eighth of the area of circle. Prove that 8 sin`theta/2 "cos"theta/2+pi =(pitheta)/45`

In the given figure, PQ and AB are respectively the arcs of two concentric circles of radii 7 cm and 3.5 cm with centre O. If ∠POQ = 30°, find the area of the shaded region.

Four cows are tethered at the four corners of a square field of side 50 m such that the each can graze the maximum unshared area. What area will be left ungrazed?
Find the area of a sector of a circle having radius 6 cm and length of the arc 15 cm.
Find the difference of the areas of a sector of angle 120° and its corresponding major sector of a circle of radius 21 cm.
