Advertisements
Advertisements
प्रश्न
Find the number of revolutions made by a circular wheel of area 1.54 m2 in rolling a distance of 176 m.
उत्तर
Let the number of revolutions made by a circular wheel be n and the radius of circular wheel be r.
Given that, area of circular wheel = 1.54 m2
⇒ πr2 = 1.54 ...[∵ Area of circular πr2]
⇒ r2 = `1.54/22 xx 7`
⇒ r2 = 0.49
∴ r = 0.7 m
So, the radius of the wheel is 0.7 m
Distance travelled by a circular wheel in one revolution = Circumference of circular wheel
= 2πr
= `2 xx 22/7 xx 0.7`
= `22/5`
= 4.4 m ...[∵ Circumference of a circle = 2πr]
Since, distance travelled by a circular wheel = 176 m
∴ Number of revolutions = `"Total distance"/"Distance in one revolution"`
= `176/4.4`
= 40
Hence, the required number of revolutions made by a circular wheel is 40.
APPEARS IN
संबंधित प्रश्न
In Fig. 9, is shown a sector OAP of a circle with centre O, containing ∠θ. AB is perpendicular to the radius OQ and meets OP produced at B. Prove that the perimeter of shaded region is
`r[tantheta+sectheta+(pitheta)/180-1]`

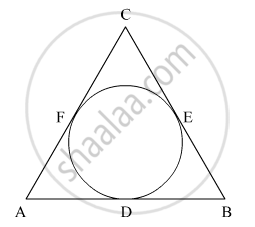
In Fig. 2, a circle is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. If the lengths of sides AB, BC and CA and 12 cm, 8 cm and 10 cm respectively, find the lengths of AD, BE and CF.
A gardener wants to fence a circular garden of diameter 21 m. Find the length of the rope he needs to purchase, if he makes 2 rounds of fence. Also find the costs of the rope, if it cost ₹ 4 per meter. (Take `pi = 22/7`)
ABC is an isosceles right-angled triangle with ∠ABC = 90°. A semi-circle is drawn with AC as the diameter. If AB = BC = 7 cm, find the area of the shaded region. [Take π = 22/7]
The areas of two circles are in the ratio 4: 9. What is the ratio between their circumferences?
The sum of the radii of two circles is 7 cm, and the difference of their circumferences is 8 cm. Find the circumference of the circles.
The sum of the radii of two circles is 10.5 cm and the difference of their circumferences is 13. 2 cm. Find the radii of the two circles.
A cylindrical beaker of 7 cm diameter is partly filled with water. Determine the number of spherical marbles of diameter 1.4 cm that are to be submerged in it to raise the water level by 5.6 cm
The area of a circle whose circumference is 22 cm, is ____________.
The numerical value of the area of a circle is greater than the numerical value of its circumference. Is this statement true? Why?
