Advertisements
Advertisements
प्रश्न
Given equation of line L1 is y = 4.
(i) Write the slope of line, if L2 is the bisector of angle O.
(ii) Write the coordinates of point P.
(iii) Find the equation of L2
उत्तर
Equation of L1 is y = 4 (given)
(i) As L2 is bisector of O
⇒ L2 is inclined at an angle of 45° with XX'
∴ Slope of L2 = m = tan 45° = 1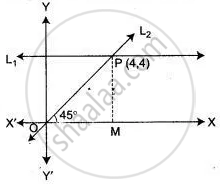
(ii) Slope of L2 = `(4 - 0)/(x - 0)`
⇒ 1 = `(4)/x`
⇒ x = 4
So coordinates of P are (4, 4).
(Since the slope of L2 is 1, L2 ⇒ PM = OM)
(iii) L2 passes through O(0, 0), P(4, 4) and has slope m = 1
∴ Equation of L2 is
y - y1 = m (x - x1)
y - 0 = 1 (x - 0)
or y = x
or x - y = 0.
APPEARS IN
संबंधित प्रश्न
State, true or false :
The point (8, 7) lies on the line y – 7 = 0
Does the line 3x − 5y = 6 bisect the join of (5, −2) and (−1, 2)?
Find the equation of the line whose slope is `-5/6` and x-intercept is 6.
Find the equations of the line through (1, 3) and making an intercept of 5 on the y-axis.
The line through P (5, 3) intersects y-axis at Q.
write the equation of the line
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the equation of a line, through the centroid and parallel to AB.
Find the value of m if the line 2x + 5y + 12 = 0 passes through the point
( 4,m ).
A(8,5), B (-2,1) and C(5,4) are the vertices of a triangle. Find the equation of the median of the traingle through C.
Find the equations of a line passing through the point (2, 3) and having the x – interecpt of 4 units.
A and B are two points on the x-axis and y-axis respectively.

- Write down the coordinates of A and B.
- P is a point on AB such that AP : PB = 1 : 1.
Using section formula find the coordinates of point P. - Find the equation of a line passing through P and perpendicular to AB.
