Question

If A(−4, 8), B(−3, −4), C(0, −5) and D(5, 6) are the vertices of a quadrilateral ABCD, find its area.

Answer 1

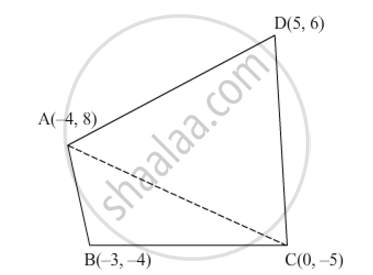
Let the vertices of the quadrilateral be A(−4, 8), B(−3, −4), C(0, −5) and D(5, 6).

Join AC to form two triangles, namely, ΔABC and ΔACD.

Area of quadrilateral ABCD = Area of ΔABC + Area of ΔACD

We know

Area of triangle having vertices (x₁, y₁), (x₂, y₂) and (x₃, y₃) =`1/2`[x₁(y₂−y₃)+x₂(y₃−y₁)+x₃(y₁−y₂)]

Now,

Area of ∆ABC=`1/2`{(−4)[(−4)−(−5)]+(−3)[(−5)−(8)]+(0)[(8)−(−4)]}

=`1/2`(−4+39)

`=35/2 `

Area of ∆ACD=`1/2`{(−4)[(−5)−(6)]+(0)[(6)−(8)]+(5)[(8)−(−5)]}

=`1/2`(44+65)

`=109/2`

∴Area of quadrilateral ABCD=Area of ∆ABC+Area of ∆ACD

`35/2+109/2`

=72 square units

Thus, the area of quadrilateral ABCD is 72 square units.