Advertisements
Advertisements
प्रश्न
If one angle of a triangle is 60° and the other two angles are in the ratio 1:2, find the angles.
उत्तर
Given, one angle of a triangle is 60°.
Let the other two angles be x and 2x.
We know that, the sum of all angles in a triangle is equal to 180°.
So, x + 2x + 60° = 180°
⇒ 3x = 180° – 60°
⇒ 3x = 120°
⇒ x = 40°
So, the other two angles will be x = 40° and 2x = 2 × 40° = 80°.
APPEARS IN
संबंधित प्रश्न
In the given figure, the side QR of ΔPQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR = 1/2∠QPR.

Find the value of the unknown x in the given diagram:
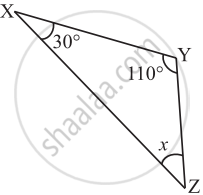
Find the value of the unknown x in the following diagram:

Find the value of the unknown x in the following diagram:
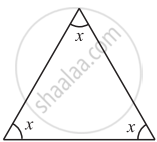
If the three angles of a triangle are in the ratio 3 : 5 : 4, then find them
Prove that a triangle must have atleast two acute angles.
It is possible to have a triangle in which two of the angles are right angles.
The angles of a triangle are in the ratio 2:3:5. Find the angles.
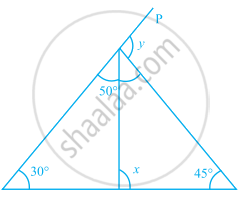
Find the values of x and y in the given figure.
In ΔPQR, if 3∠P = 4∠Q = 6∠R, calculate the angles of the triangle.
