Advertisements
Advertisements
प्रश्न
In an isosceles triangle, one angle is 70°. The other two angles are of ______.
- 55° and 55°
- 70° and 40°
- any measure
In the given option(s) which of the above statement(s) are true?
पर्याय
(i) only
(ii) only
(iii) only
(i) and (ii)
उत्तर
(i) and (ii)
Explanation:
From the question, it is given that,
One angle of an isosceles triangle is 70o.
We know that, in an isosceles triangle 2 angles are equal corresponding with 2 equal sides,
If 70° is 3rd angle of triangle,
70° + x + x = 180o
2x + 70° = 180o
2x = 180° – 70o
x = `110^circ/2`
x = 55°
So, both angles are 55°
Consider the 70° as base angle of an isosceles triangle
Then, 70° + 70° + x = 180°
x = 180° – 140°
x = 40°
So, one angle is 40° and another is 70°.
APPEARS IN
संबंधित प्रश्न
Find the value of the unknown x in the following diagram:

Find the value of the unknown x and y in the following diagram:
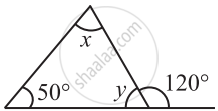
Find the value of the unknown x and y in the following diagram:

In ∆DEF, ∠F = 48°, ∠E = 68° and bisector of ∠D meets FE at G. Find ∠FGD
How many triangles can be drawn having its angles as 53°, 64° and 63°? Give reason for your answer.
In the following figure, ∠Q > ∠R, PA is the bisector of ∠QPR and PM ⊥ QR. Prove that ∠APM = `1/2` (∠Q – ∠R).
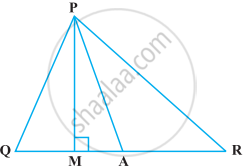
It is possible to have a triangle in which each angle is less than 60°.
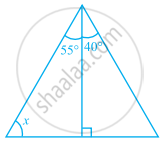
In the given figure, find the value of x.
The angles of a triangle are arranged in descending order of their magnitudes. If the difference between two consecutive angles is 10°, find the three angles.
If one angle of a triangle is 60° and the other two angles are in the ratio 1:2, find the angles.
