Advertisements
Advertisements
प्रश्न
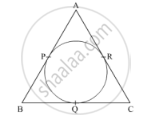
In Fig. 1, the sides AB, BC and CA of a triangle ABC, touch a circle at P, Q and R respectively. If PA = 4 cm, BP = 3 cm and AC = 11 cm, then the length of BC (in cm) is ?
पर्याय
A. 11
B. 10
C. 14
D. 15
उत्तर
Given, AP = 4 cm, BP = 3 cm and AC = 11 cm.
The lengths of tangents drawn from an external point to the circle are equal.
AP = AR, BP = BQ, CQ = CR …….. (1)
AC = 11 cm
⇒ AR + RC = 11 cm
⇒ AP + CQ = 11 cm [From equation (1)]
⇒ 4 cm + CQ = 11 cm
⇒ CQ = (11 − 4) cm
⇒ CQ = 7 cm
BP = BQ = 3 cm
Now, BC = BQ + QC
⇒ BC = (3 + 7) cm
⇒ BC = 10 cm
Hence, the correct option is B.
APPEARS IN
संबंधित प्रश्न
The lengths of three consecutive sides of a quadrilateral circumscribing a circle are 4cm,5cm and 7cm respectively. Determine the length of fourth side.
In the given figure, O is the centre of the circle. If ∠AOB = 140° and ∠OAC = 50°; find:
- ∠ACB,
- ∠OBC,
- ∠OAB,
- ∠CBA.

In the given figure, PA and PB are two tangents to the circle with centre O. If ∠APB = 60° then find the measure of ∠OAB.

One chord of a circle is known to be 10 cm. The radius of this circle must be
ABC is a triangle with B as right angle, AC = 5 cm and AB = 4 cm. A circle is drawn with Aas centre and AC as radius. The length of the chord of this circle passing through C and B is
Number of circles that can be drawn through three non-collinear points is
In the given figure, O is the centre of the circle and ∠BDC = 42°. The measure of ∠ACB is

Construct a triangle ABC with AB = 4.2 cm, BC = 6 cm and AC = 5cm. Construct the circumcircle of the triangle drawn.
AD is a diameter of a circle and AB is a chord. If AD = 34 cm, AB = 30 cm, the distance of AB from the centre of the circle is ______.
In the given figure, O is the centre of the circle. If ∠ AOB = 145°, then find the value of x.

