Advertisements
Advertisements
प्रश्न
In the given figure, AB || DE. Find ∠ACD.

उत्तर
In the given problem, AB || DE
We need to find ∠ACD
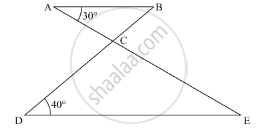
Now, AB || DE and AEis the transversal, so using the property, “alternate interior angles are equal”, we get,
∠BAE = ∠AED
∠AED = 30
Further, applying angle sum property of the triangle
In ΔDCE
∠DCE + ∠D + ∠E = 180
∠DCE + 40 + 30 = 180
∠DCE + 70 = 180
∠DCE = 180° - 70°
∠DCE = 110°
Further, ACE is a straight line, so using the property, “the angles forming a linear pair are supplementary”, we get,
∠ACD + ∠DCE = 180°
∠ACD + 110 = 180°
∠ACD = 180 - 110°
∠ACD = 70°
Therefore, ∠ACD = 70° .
APPEARS IN
संबंधित प्रश्न
The angles of a triangle are (x − 40)°, (x − 20)° and `(1/2x-10)^@.` find the value of x
Is the following statement true and false :
An exterior angle of a triangle is less than either of its interior opposite angles.
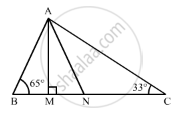
In the given figure, AM ⊥ BC and AN is the bisector of ∠A. If ∠B = 65° and ∠C = 33°, find ∠MAN.
The bisects of exterior angle at B and C of ΔABC meet at O. If ∠A = x°, then ∠BOC =
State, if the triangle is possible with the following angles :
60°, 60°, and 50°
Calculate the unknown marked angles of the following figure :
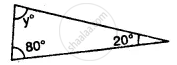
In the following, find the marked unknown angle:

The length of the three segments is given for constructing a triangle. Say whether a triangle with these sides can be drawn. Give the reason for your answer.
12 cm, 12 cm, 16 cm
O is a point in the interior of a square ABCD such that OAB is an equilateral triangle. Show that ∆OCD is an isosceles triangle.
Identify three triangles in the figure.