Question

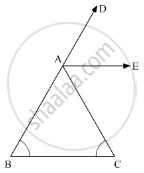
In the given figure, AE bisects ∠CAD and ∠B= ∠C. Prove that AE || BC.

Answer 1

In the given problem, AE bisects ∠CAD and ∠B = ∠C

We need to prove AE || BC

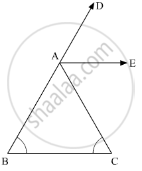
As, ∠CAD  is bisected by AE

 ∠CAD = 2∠CAE - 2∠DAE         ..........(1)

Now, using the property, “an exterior angle of a triangle in equal to the sum of the two opposite interior angles”, we get,

 ∠CAD ∠B +∠C

∠CAD = 2∠C (∠B = ∠C)

 2∠CAE = 2∠C (using 1)

∠CAE = ∠C

∠CAE = ∠ACB

Hence, using the property, if alternate interior angles are equal, then the two lines are parallel, we get,

∠CAE = ∠ACB

Thus,AE || BC

Hence proved.