Advertisements
Advertisements
प्रश्न
In an isosceles triangle, if the vertex angle is twice the sum of the base angles, then the measure of vertex angle of the triangle is
पर्याय
100°
120°
110°
130°
उत्तर
Let ABCbe isosceles triangle
Then
AB = AC
∠B = ∠C
Now it is given that vertex angle is 2 times the sum of base angles
⇒∠A = 2 ∠(B+ C)
⇒∠A = 2 ∠(∠B + ∠B ) (As ∠B = ∠C)
⇒∠A = 2 (2∠B)
⇒∠A = 4 ∠B
Now
∠A + ∠B + ∠C = 180° (Property of triangle)
4∠B + ∠B + ∠B = 180° (Since ∠A = 4∠B, and ∠B = ∠C )
6∠B = 180°
∠B = 30°
∠A = 4∠B
`= 4 xx 30°`
= 120°
APPEARS IN
संबंधित प्रश्न
In a squared sheet, draw two triangles of equal areas such that
The triangles are congruent.
What can you say about their perimeters?
In the following example, a pair of triangles is shown. Equal parts of triangle in each pair are marked with the same sign. Observe the figure and state the test by which the triangle in each pair are congruent.
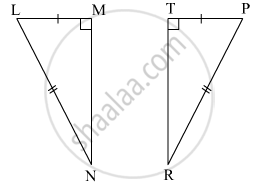
By ______ test
ΔLMN ≅ ΔPTR
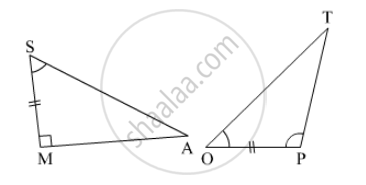
Observe the information shown in pair of triangle given below. State the test by which the two triangles are congruent. Write the remaining congruent parts of the triangles.

From the information shown in the figure,
in ΔPTQ and ΔSTR
seg PT ≅ seg ST
∠PTQ ≅ ∠STR ...[Vertically opposite angles]
∴ ΔPTQ ≅ ΔSTR ...`square` test
∴ `{:("∠TPQ" ≅ square),("and" square ≅ "∠TRS"):}}` ...corresponding angles of congruent triangles
seg PQ ≅ `square` ...corresponding sides of congruent triangles
In the pair of triangles in the following figure, parts bearing identical marks are congruent. State the test and the correspondence of vertices by the triangle in pairs is congruent.
On the sides AB and AC of triangle ABC, equilateral triangle ABD and ACE are drawn. Prove that:
- ∠CAD = ∠BAE
- CD = BE
In the following figure, OA = OC and AB = BC.
Prove that:
(i) ∠AOB = 90o
(ii) ΔAOD ≅ ΔCOD
(iii) AD = CD
In the following figure, OA = OC and AB = BC.

Prove that: AD = CD
Two right-angled triangles ABC and ADC have the same base AC. If BC = DC, prove that AC bisects ∠BCD.
Which of the following rule is not sufficient to verify the congruency of two triangles
If AB = QR, BC = PR and CA = PQ, then ______.
