Advertisements
Advertisements
प्रश्न
In square PQRS :
(i) if PQ = 3x – 7 and QR = x + 3 ; find PS
(ii) if PR = 5x and QS = 9x – 8. Find QS
उत्तर
(i) sides of square are equal.
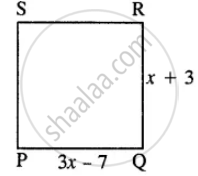
PQ = QR
⇒ 3x – 7 = x + 3
⇒ 3x – x = 3 + 7
⇒ 2x = 10
x = 5
PS=PQ = 3x – 7 = 3 x 5 – 7 =8
(ii) PR = 5x and QS = 9x – 8
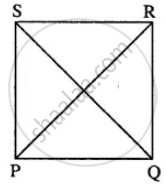
As diagonals of square are equal.
PR = QS
5x = 9x – 8
⇒ 5x – 9x = -8
⇒ -4x = -8
⇒ x = 2
QS = 9x – 8 = 9 x 2 – 8 =10
APPEARS IN
संबंधित प्रश्न
All squares are rhombuses and also rectangles.
All squares are not parallelograms.
Identify all the quadrilaterals that have four right angles
Explain how a square is a quadrilateral
Explain how a square is a parallelogram
Explain how a square is a rhombus.
In a quadrilateral ABCD, AB = AD and CB = CD.
Prove that:
- AC bisects angle BAD.
- AC is the perpendicular bisector of BD.
ABCD is a square. A is joined to a point P on BC and D is joined to a point Q on AB. If AP = DQ;
prove that AP and DQ are perpendicular to each other.
PQRS is a square whose diagonals PR and QS intersect at O.M is a point on QR such that OQ = MQ. Find the measures of ∠MOR and ∠QSR.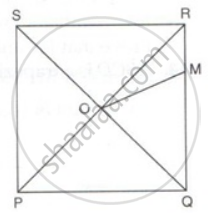
In a parallelogram PQRS, T is any point on the diagonal PR. If the area of ΔPTQ is 18 square units find the area of ΔPTS.
