Advertisements
Advertisements
प्रश्न
In a quadrilateral ABCD, AB = AD and CB = CD.
Prove that:
- AC bisects angle BAD.
- AC is the perpendicular bisector of BD.
Advertisements
उत्तर
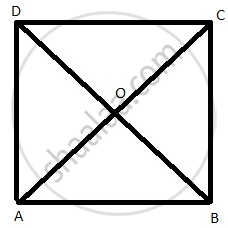
Given: ABCD is quadrilateral,
AB = AD
CB = CD
To prove:
- AC bisects angle BAD.
- AC is the perpendicular bisector of BD.
Proof:
In ΔABC and ΔADC,
AB = AD ...(given)
CB = CD ...(given)
AC = AC ...(Common side)
ΔABC ≅ ΔADC ...(SSS)
∠BAD = ∠DAO ...(AC bisects A)
Therefore, AC bisects ∠BAD
OD = OB
OA = OC ...(diagonals bisect each other at O)
Thus, AC is perpendicular bisector of BD.
Hence, proved.
APPEARS IN
संबंधित प्रश्न
All squares are not parallelograms.
Identify all the quadrilaterals that have four right angles
Explain how a square is a parallelogram
Explain how a square is a rhombus.
Prove that the bisectors of the interior angles of a rectangle form a square.
PQRS is a square whose diagonals PR and QS intersect at O.M is a point on QR such that OQ = MQ. Find the measures of ∠MOR and ∠QSR.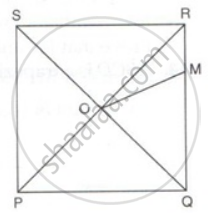
In the given figure, ΔPQR is right-angled at P. PABQ and QRST are squares on the side PQ and hypotenuse QR. If PN ⊥ TS, show that:
(a) ΔQRB ≅ ΔPQT
(b) Area of square PABQ = area of rectangle QTNM.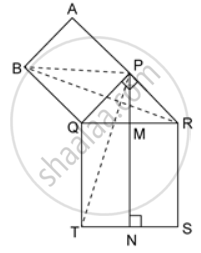
In the given figure AF = BF and DCBF is a parallelogram. If the area of ΔABC is 30 square units, find the area of the parallelogram DCBF.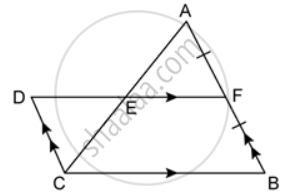
In a parallelogram PQRS, M and N are the midpoints of the sides PQ and PS respectively. If area of ΔPMN is 20 square units, find the area of the parallelogram PQRS.
In a parallelogram PQRS, T is any point on the diagonal PR. If the area of ΔPTQ is 18 square units find the area of ΔPTS.
