Advertisements
Advertisements
प्रश्न
In the given figure AF = BF and DCBF is a parallelogram. If the area of ΔABC is 30 square units, find the area of the parallelogram DCBF.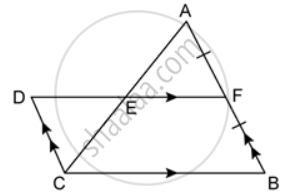
उत्तर
In ΔABC,
AF = FB and EF || BC ...(given)
∴ AE = EC ...(Converse of Midpoint theorem) ...(i)
In ΔAEF and ΔCED,
∠FEA = ∠DEC ...(Vertically opposite angles)
CE = AE ...[From (i)]
∠FAE = ∠DCE ...(Alternate angles)
∴ ΔFAE ≅ ΔCED ...( ASA test of congruency)
⇒ A(ΔAEF) = A(ΔCED) ....(ii)
A(ΔABC)
= A(ΔAEF) + A(EFBC)
= A(ΔCED) + A(EFBC) ....[From (ii)]
∴ A(ΔABC) = A(||gm DCBF)
⇒ A(||gm DCBF) = 30 sq. units.
APPEARS IN
संबंधित प्रश्न
All rectangles are squares
All squares are rhombuses and also rectangles.
All squares are not parallelograms.
Identify all the quadrilaterals that have four right angles
Explain how a square is a parallelogram
In a quadrilateral ABCD, AB = AD and CB = CD.
Prove that:
- AC bisects angle BAD.
- AC is the perpendicular bisector of BD.
Prove that the bisectors of the interior angles of a rectangle form a square.
ABCD is a square. A is joined to a point P on BC and D is joined to a point Q on AB. If AP = DQ;
prove that AP and DQ are perpendicular to each other.
Prove that the quadrilateral formed by joining the mid-points of a square is also a square.
In a parallelogram PQRS, M and N are the midpoints of the sides PQ and PS respectively. If area of ΔPMN is 20 square units, find the area of the parallelogram PQRS.
