Advertisements
Advertisements
Question
In square PQRS :
(i) if PQ = 3x – 7 and QR = x + 3 ; find PS
(ii) if PR = 5x and QS = 9x – 8. Find QS
Solution
(i) sides of square are equal.
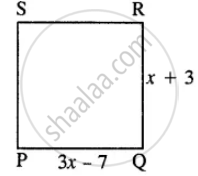
PQ = QR
⇒ 3x – 7 = x + 3
⇒ 3x – x = 3 + 7
⇒ 2x = 10
x = 5
PS=PQ = 3x – 7 = 3 x 5 – 7 =8
(ii) PR = 5x and QS = 9x – 8
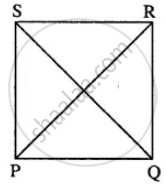
As diagonals of square are equal.
PR = QS
5x = 9x – 8
⇒ 5x – 9x = -8
⇒ -4x = -8
⇒ x = 2
QS = 9x – 8 = 9 x 2 – 8 =10
APPEARS IN
RELATED QUESTIONS
All squares are rhombuses and also rectangles.
All squares are not parallelograms.
Identify all the quadrilaterals that have four right angles
Explain how a square is a rhombus.
Explain how a square is a rectangle
Prove that the bisectors of the interior angles of a rectangle form a square.
Prove that the quadrilateral formed by joining the mid-points of a square is also a square.
In the given figure, ΔPQR is right-angled at P. PABQ and QRST are squares on the side PQ and hypotenuse QR. If PN ⊥ TS, show that:
(a) ΔQRB ≅ ΔPQT
(b) Area of square PABQ = area of rectangle QTNM.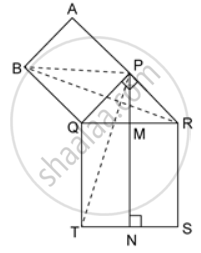
In the given figure AF = BF and DCBF is a parallelogram. If the area of ΔABC is 30 square units, find the area of the parallelogram DCBF.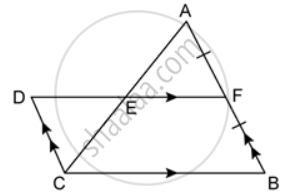
In a parallelogram PQRS, T is any point on the diagonal PR. If the area of ΔPTQ is 18 square units find the area of ΔPTS.
