Advertisements
Advertisements
प्रश्न
In the adjacent figure ∠BAC = 90° and AD ⊥ BC then

पर्याय
BD.CD = BC2
AB.AC = BC2
BD.CD = AD2
AB.AC = AD2
उत्तर
BD.CD = AD2
Explanation;
Hint: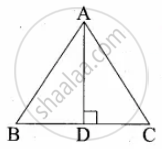
ΔBAC ∼ ΔBDA
`"AC"/"AD" = "AB"/"BD"` ...(1)
ΔCAB ∼ ΔCDA
`"AC"/"CD" = "AB"/"AD"` ...(2)
From (1) and (2) we get
`"BD"/"AD" = "AD"/"CD"`
AD2 = BD·CD
APPEARS IN
संबंधित प्रश्न
The diagonal BD of a parallelogram ABCD intersects the segment AE at the point F, where E is any point on the side BC. Prove that DF × EF = FB × FA
In the given figure, if ∠ADE = ∠B, show that ΔADE ~ ΔABC. If AD = 3.8cm, AE = 3.6cm, BE = 2.1cm and BC = 4.2cm, find DE.
ΔABC is right angled at A and AD⊥BC. If BC = 13cm and AC = 5cm, find the ratio of the areas of ΔABC and ΔADC.
In Δ ABC, DE || BC; DC and EB intersects at F. if `"DE"/"BC" = 2/7` , find `("Ar" (triangle "FDE"))/("Ar" (triangle "FBC"))`
Δ ABC ∼ Δ PQR such that AB= 1.5 cm and PQ=2. 1 cm. Find the ratio of areas of Δ ABC and ΔPQR.
A triangle ABC is enlarged, about the point O as centre of enlargement, and the scale factor is 3. Find : OA, if OA' = 6 cm.
A model of cargo tuck is made to a scale of 1:40. The length of the model is 15cm. Calculate: The length of the truck
Two vertical poles of heights 6 m and 3 m are erected above a horizontal ground AC. Find the value of y

If ∆ABC is an isosceles triangle with ∠C = 90° and AC = 5 cm, then AB is
From the given figure, prove that ΔABC ~ ΔEDF
