Advertisements
Advertisements
प्रश्न
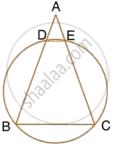
In the given figure, AB = AC. Prove that DECB is an isosceles trapezium.
उत्तर

Here, AB = AC
`=>` ∠B = ∠C
∴ DECB is a cyclic quadrilateral
(In a triangle, angles opposite to equal sides are equal)
Also, ∠B + ∠DEC = 180° ...(1)
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
`=>` ∠C + ∠DEC = 180° ...[From (1)]
But this is the sum of interior angles
On one side of a transversal.
∴ DE || BC
But ∠ADE = ∠AED = ∠C ...[Corresponding angles]
Thus, ∠ADE = ∠AED
`=>` AD = AE
`=>` AB – AD = AC – AE ...(∴ AB = AC)
`=>` BD = CE
Thus, we have, DE || BC and BD = CE
Hence, DECB is an isosceles trapezium
APPEARS IN
संबंधित प्रश्न
ABCD is a cyclic quadrilateral in a circle with centre O. If ∠ADC = 130°; find ∠BAC.

In a cyclic quadrilateral ABCD, the diagonal AC bisects the angle BCD. Prove that the diagonal BD is parallel to the tangent to the circle at point A.
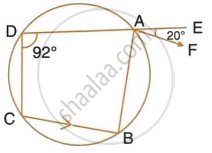
In the given figure, ABCD is a cyclic quadrilateral. AF is drawn parallel to CB and DA is produced to point E. If ∠ADC = 92°, ∠FAE = 20°; determine ∠BCD. Give reason in support of your answer.
Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of Δ DEF are 90° - `"A"/2` , 90° - `"B"/2` and 90° - `"C"/2` respectively.
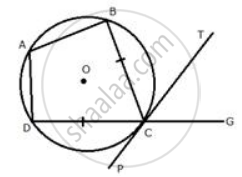
In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If angle BCG=108° and O is the centre of the circle, find: angle DOC
ABCD is a cyclic quadrilateral AB and DC are produced to meet in E. Prove that Δ EBC ∼ Δ EDA.
Prove that the quadrilateral formed by angle bisectors of a cyclic quadrilateral ABCD is also cyclic.
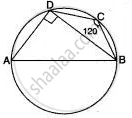
In the adjoining figure, AB is the diameter of the circle with centre O. If ∠BCD = 120°, calculate:
(i) ∠BAD (ii) ∠DBA
In the given circle with centre O, ∠ABC = 100°, ∠ACD = 40° and CT is a tangent to the circle at C. Find ∠ADC and ∠DCT.

An exterior angle of a cyclic quadrilateral is congruent to the angle opposite to its adjacent interior angle, to prove the theorem complete the activity.
Given: ABCD is cyclic,
`square` is the exterior angle of ABCD
To prove: ∠DCE ≅ ∠BAD
Proof: `square` + ∠BCD = `square` .....[Angles in linear pair] (I)
ABCD is a cyclic.
`square` + ∠BAD = `square` ......[Theorem of cyclic quadrilateral] (II)
By (I) and (II)
∠DCE + ∠BCD = `square` + ∠BAD
∠DCE ≅ ∠BAD
