Advertisements
Advertisements
प्रश्न
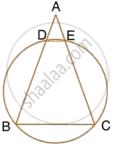
In the given figure, AB = AC. Prove that DECB is an isosceles trapezium.
उत्तर

Here, AB = AC
`=>` ∠B = ∠C
∴ DECB is a cyclic quadrilateral
(In a triangle, angles opposite to equal sides are equal)
Also, ∠B + ∠DEC = 180° ...(1)
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
`=>` ∠C + ∠DEC = 180° ...[From (1)]
But this is the sum of interior angles
On one side of a transversal.
∴ DE || BC
But ∠ADE = ∠AED = ∠C ...[Corresponding angles]
Thus, ∠ADE = ∠AED
`=>` AD = AE
`=>` AB – AD = AC – AE ...(∴ AB = AC)
`=>` BD = CE
Thus, we have, DE || BC and BD = CE
Hence, DECB is an isosceles trapezium
APPEARS IN
संबंधित प्रश्न
In the figure given, O is the centre of the circle. ∠DAE = 70°. Find giving suitable reasons, the measure of:
- ∠BCD
- ∠BOD
- ∠OBD

In the figure, given below, find:
- ∠BCD,
- ∠ADC,
- ∠ABC.
Show steps of your working.

ABCD is a cyclic quadrilateral in a circle with centre O. If ∠ADC = 130°; find ∠BAC.

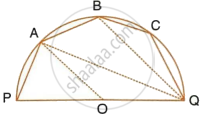
The given figure shows a semi-circle with centre O and diameter PQ. If PA = AB and ∠BCQ =140°; find measures of angles PAB and AQB. Also, show that AO is parallel to BQ.
In a cyclic quadrialteral ABCD , if m ∠ A = 3 (m ∠C). Find m ∠ A.
In a circle with centre O , chords AB and CD intersets inside the circle at E . Prove that ∠ AOC = ∠ BOD = 2 ∠ AEC.
ABCD is a parallelogram. A circle through vertices A and B meets side BC at point P and side AD at point Q. Show that quadrilateral PCDQ is cyclic.
If ABCD is a cyclic quadrilateral in which AD || BC. Prove that ∠B = ∠C.
The diagonals of a cyclic quadrilateral are at right angles. Prove that the perpendicular from the point of their intersection on any side when produced backward bisects the opposite side.
In the given circle with centre O, ∠ABC = 100°, ∠ACD = 40° and CT is a tangent to the circle at C. Find ∠ADC and ∠DCT.

