Advertisements
Advertisements
प्रश्न
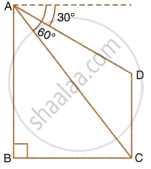
In the given figure, from the top of a building AB = 60 m hight, the angle of depression of the top and bottom of a vertical lamp post CD are observed to be 30° and 60° respectively. Find:
- the horizontal distance between AB and CD.
- the height of the lamp post.
उत्तर
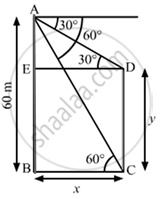
Given that AB is a building that is 60 m, high.
Let BC = DE = x and CD = BE = y
`=>` AE = AB – BE = 60 – y
i. In right ΔAED,
`tan 30^circ = (AE)/(DE)`
`=> 1/sqrt(3) = (60 - y)/(x)`
`=> x = 60sqrt(3) - ysqrt(3)` ...(1)
In right ΔABC,
`=> tan 60^circ = (AB)/(BC)`
`=> sqrt(3) = 60/x`
`=> x = 60/sqrt(3)`
`=> x = 60/sqrt(3) xx sqrt(3)/sqrt(3)`
`=> x = (60sqrt(3))/3`
`=> x = 20sqrt(3)`
`=>` x = 20 × 1.732
`=>` x = 34.64 m
Thus, the horizontal distance between AB and CD is 34.64 m.
ii. From (1), we get the height of the lamp post = CD = y
`x = 60sqrt(3) - ysqrt(3)`
`=> 20sqrt(3) = 60sqrt(3) - ysqrt(3)`
`=>` 20 = 60 – y
`=>` y = 60 – 20
`=>` y = 40 m
Thus, the height of the lamp post is 40 m.
APPEARS IN
संबंधित प्रश्न
A kite is attached to a string. Find the length of the string, when the height of the kite is 60 m and the string makes an angle 30° with the ground.
Find the height of a building, when it is found that on walking towards it 40 m in a horizontal line through its base the angular elevation of its top changes from 30° to 45°.
Calculate AB.
At a point on level ground, the angle of elevation of a vertical tower is found to be such that its tangent is `5/12`. On walking 192 metres towards the tower, the tangent of the angle is found to be `3/4`. Find the height of the tower.
A vertical tower stand on horizontel plane and is surmounted by a vertical flagstaff of height h metre. At a point on the plane, the angle of elevation of the bottom of the flagstaff is α and that of the top of flagstaff is β. Prove that the height of the tower is
`(h tan alpha)/(tan beta - tan alpha)`
The angle of elevation of the top of a vertical cliff from a point 30 m away from the foot of the cliff is 60°. Find the height of the cliff.
An aeroplane takes off at angle of `30^circ` with the ground . Find the height of the aeroplane above the ground when it has travelled 386m without changing direction .
A man in a boat rowing away from a lighthouse 180 m high takes 2 minutes to change the angle of elevation of the top of the lighthouse from 60° and 30°. Find the speed of the boat.
If the angle of elevation of a cloud from a point h m above a lake is α, and the angle of depression of its reflection in the lake be β, prove that distance of the cloud from the point of observation is `("2h"secα)/(tanα - tanβ)`.
Two men on either side of a temple 75 m high observed the angle of elevation of the top of the temple to be 30° and 60° respectively. Find the distance between the two men.
