Question

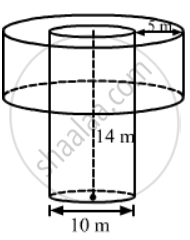
In a village, a well with 10 m inside diameter, is dug 14 m deep. Earth taken out of it is spread all around to a width 5 m to form an embankment. Find the height of the embankment. What value of the villagers is reflected here? 

Answer 1

We have, 

Radius of well, R = `10/2` = 5 m,

Depth of the well, H = 14 m and 

Width of the embarkment = 5 m,

Also, the outer radius of the embankment, r = R + 5 = 5 + 5 = 10 m

And , the inner radius of the embarkment = R = 5m

Let the height of the embankment = 5 m,

Also, the outer radius of the embankment, r = R + 5 = 5 + 5 = 10 m 

And, the inner radius of the embankment = R = 5 m

Let the height of the embarkment be h.

Now,

Volume of the embankment = Volume of the earth taken out 

⇒  Volume of embankment = Volume of the well

⇒ (πr² - πR²) h = πR²H

⇒ π (r² - R²) h = πR²H  

⇒ (r² - R²) h = R²H

⇒ (10² - 5²) h = 5 × 5 × 14

⇒ (100 - 25) h = 25 × 14

⇒ 75 h = 25 × 14

`⇒ "h" = (25xx14)/75 `

`therefore  "h" = 14/3  "m"`

So, the height of the embankment is `14/3` m

Value: We must lanour hard to make maximum use of the available resources.

Disclaimer: The answer provided in the textbook is incorrect. It has been corrected above.