Advertisements
Advertisements
प्रश्न
Prove that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
उत्तर

Given: PQ is a diameter of circle which Bisects
Chord AB at C
To prove: PQ bisects ∠AOB
Proof:
In ΔAOC and ΔBOC
OA=OB
OC=OC
AC=BC
Then Δ AOC ≅ BOC
∴∠AOC = ∠BOC
Hence PQ bisects ∠AOB
APPEARS IN
संबंधित प्रश्न
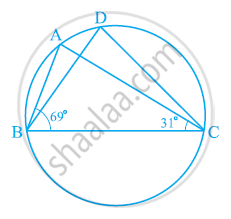
In the given figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.
Given an arc of a circle, complete the circle.
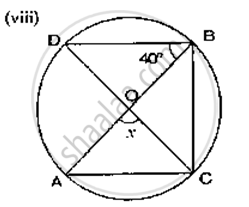
If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure
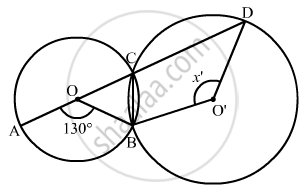
In the given figure, O and O' are centres of two circles intersecting at B and C. ACD is a straight line, find x.
In the given figure, AB is a diameter of the circle such that ∠A = 35° and ∠Q = 25°, find ∠PBR.

In the given figure, P and Q are centres of two circles intersecting at B and C. ACD is a straight line. Then, ∠BQD =

If the given figure, AOC is a diameter of the circle and arc AXB = \[\frac{1}{2}\] arc BYC. Find ∠BOC.
A chord of a circle is equal to its radius. Find the angle subtended by this chord at a point in major segment.
A circle has radius `sqrt(2)` cm. It is divided into two segments by a chord of length 2 cm. Prove that the angle subtended by the chord at a point in major segment is 45°.
