Advertisements
Advertisements
प्रश्न
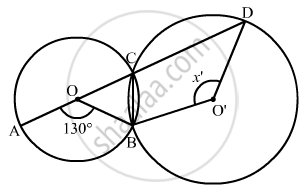
In the given figure, O and O' are centres of two circles intersecting at B and C. ACD is a straight line, find x.
उत्तर
It is given that
Two circles having center O and O' and ∠AOB = 130°
And AC is diameter of circle having center O
We have
`angle ACB =1/2 angleAOB = 65°`
So
`angleDCB = 180° - angleACB`
= 180° - 65°
= 115°
Now, reflex `angleBO'D = 2 angleBCD`
So
`360° - x° = 2 xx 115 `
= 230°
APPEARS IN
संबंधित प्रश्न
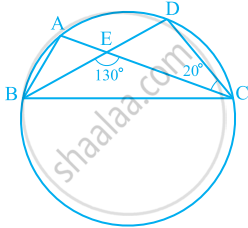
In the given figure, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°. Find ∠BAC.
Fill in the blank:
A circle divides the plane, on which it lies, in ............ parts.
Prove that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
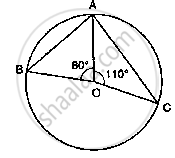
In the below fig. O is the centre of the circle. Find ∠BAC.
If O is the centre of the circle, find the value of x in the following figure

Prove that the line segment joining the mid-point of the hypotenuse of a right triangle to its opposite vertex is half the hypotenuse.
In the given figure, A is the centre of the circle. ABCD is a parallelogram and CDE is a straight line. Find ∠BCD : ∠ABE.

In the given figure, P and Q are centres of two circles intersecting at B and C. ACD is a straight line. Then, ∠BQD =

If the given figure, AOC is a diameter of the circle and arc AXB = \[\frac{1}{2}\] arc BYC. Find ∠BOC.
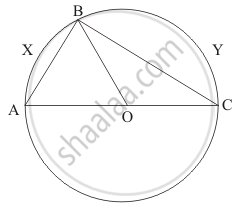
In the following figure, ∠ACB = 40º. Find ∠OAB.

