Advertisements
Advertisements
प्रश्न
A chord of a circle is equal to its radius. Find the angle subtended by this chord at a point in major segment.
Advertisements
उत्तर
Given, AB is a chord of a circle, which is equal to the radius of the circle,
i.e., AB = BO ...(i)
Join OA, AC and BC.
Since, OA = OB = Radius of circle
OA = AS = BO
Thus, ΔOAB is an equilateral triangle.
⇒ ∠AOB = 60° ...[Each angle of an equilateral triangle is 60°]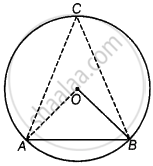
By using the theorem, in a circle, the angle subtended by an arc at the centre is twice the angle subtended by it at the remaining part of the circle.
i.e., ∠AOB = 2∠ACB
⇒ ∠ACB = `60^circ/2` = 30°
APPEARS IN
संबंधित प्रश्न
If O is the centre of the circle, find the value of x in the following figure
If O is the centre of the circle, find the value of x in the following figure
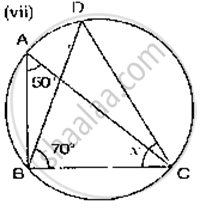
If O is the centre of the circle, find the value of x in the following figures.
Prove that the angle in a segment shorter than a semicircle is greater than a right angle.
In the given figure, two congruent circles with centres O and O' intersect at A and B. If ∠AOB = 50°, then find ∠APB.
In the given figure, if ∠AOB = 80° and ∠ABC = 30°, then find ∠CAO.
In the given figure, A is the centre of the circle. ABCD is a parallelogram and CDE is a straight line. Find ∠BCD : ∠ABE.
If arcs AXB and CYD of a circle are congruent, find the ratio of AB and CD.
In the following figure, AB and CD are two chords of a circle intersecting each other at point E. Prove that ∠AEC = `1/2` (Angle subtended by arc CXA at centre + angle subtended by arc DYB at the centre).
A circle has radius `sqrt(2)` cm. It is divided into two segments by a chord of length 2 cm. Prove that the angle subtended by the chord at a point in major segment is 45°.
