Advertisements
Advertisements
प्रश्न
If O is the centre of the circle, find the value of x in the following figure:

उत्तर
∠AOC= 135°
∴∠AOC+ ∠BOC -180°
⇒ 135° + ∠BOC = 180°
⇒∠BOC = 180° -135° = 45°
By degree measures theorem
∠BOC = 2∠CDB
⇒ 45° = 2x
`⇒x=(45°)/2=22(1°)/2.`
APPEARS IN
संबंधित प्रश्न
Prove that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
Given an arc of a circle, show how to complete the circle.
In the below fig. O is the centre of the circle. Find ∠BAC.
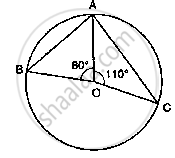
If O is the centre of the circle, find the value of x in the following figure
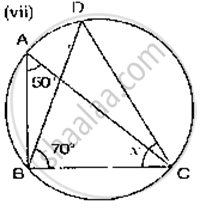
If O is the centre of the circle, find the value of x in the following figure
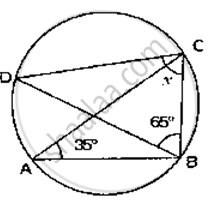
In the given figure, if ∠ACB = 40°, ∠DPB = 120°, find ∠CBD.
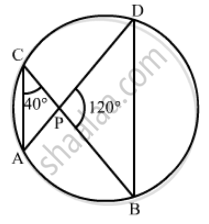
Prove that the angle in a segment greater than a semi-circle is less than a right angle.
The chord of a circle is equal to its radius. The angle subtended by this chord at the minor arc of the circle is
In the following figure, ∠ACB = 40º. Find ∠OAB.

A circle has radius `sqrt(2)` cm. It is divided into two segments by a chord of length 2 cm. Prove that the angle subtended by the chord at a point in major segment is 45°.
