Advertisements
Advertisements
प्रश्न
If O is the centre of the circle, find the value of x in the following figure:

उत्तर
∠AOC= 135°
∴∠AOC+ ∠BOC -180°
⇒ 135° + ∠BOC = 180°
⇒∠BOC = 180° -135° = 45°
By degree measures theorem
∠BOC = 2∠CDB
⇒ 45° = 2x
`⇒x=(45°)/2=22(1°)/2.`
APPEARS IN
संबंधित प्रश्न
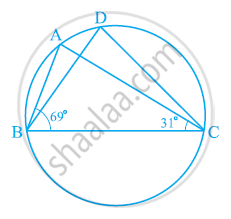
In the given figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.
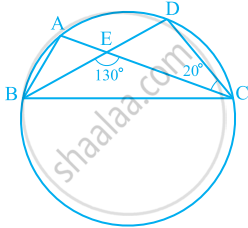
In the given figure, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°. Find ∠BAC.
Fill in the blank:
Segment of a circle is the region between an arc and .................. of the circle.
If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure

In a circle, the major arc is 3 times the minor arc. The corresponding central angles and the degree measures of two arcs are
If ABC is an arc of a circle and ∠ABC = 135°, then the ratio of arc \[\stackrel\frown{ABC}\] to the circumference is ______.
If arcs AXB and CYD of a circle are congruent, find the ratio of AB and CD.
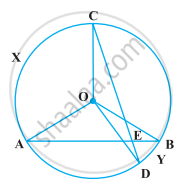
In the following figure, AB and CD are two chords of a circle intersecting each other at point E. Prove that ∠AEC = `1/2` (Angle subtended by arc CXA at centre + angle subtended by arc DYB at the centre).
A circle has radius `sqrt(2)` cm. It is divided into two segments by a chord of length 2 cm. Prove that the angle subtended by the chord at a point in major segment is 45°.
