Advertisements
Advertisements
प्रश्न
In the given figure, it is given that O is the centre of the circle and ∠AOC = 150°. Find ∠ABC.

उत्तर
It is given that O is the centre of circle and A, B and C are points on circumference.
`angle AOC = 150°` (Given)
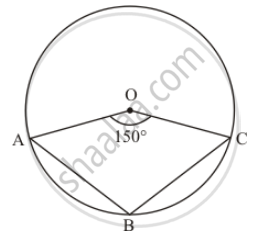
We have to find ∠ABC
The angle subtended by an arc of a circle at the centre is double the angle subtended by it at any point on the remaining part of the circle.
\[\angle ABC = \frac{1}{2}\left( \text{ reflex } \angle AOC \right)\]
\[ = \frac{1}{2}\left( 360° - 150° \right)\]
\[ = \frac{1}{2} \times 210° \]
\[ = 105° \]
Hence,`angle ABC = 105°`
APPEARS IN
संबंधित प्रश्न
Given an arc of a circle, complete the circle.
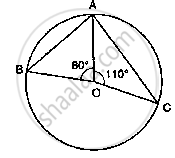
In the below fig. O is the centre of the circle. Find ∠BAC.
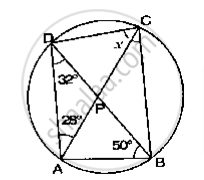
If O is the centre of the circle, find the value of x in the following figure:

If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure
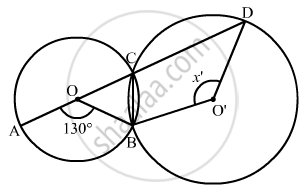
In the given figure, O and O' are centres of two circles intersecting at B and C. ACD is a straight line, find x.
Prove that the angle in a segment greater than a semi-circle is less than a right angle.
In the given figure, two congruent circles with centres O and O' intersect at A and B. If ∠AOB = 50°, then find ∠APB.

In the following figure, ∠ACB = 40º. Find ∠OAB.

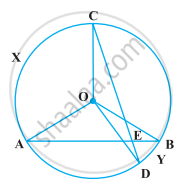
In the following figure, AB and CD are two chords of a circle intersecting each other at point E. Prove that ∠AEC = `1/2` (Angle subtended by arc CXA at centre + angle subtended by arc DYB at the centre).