Advertisements
Advertisements
प्रश्न
Prove that the intercept of a tangent between two parallel tangents to a circle subtends a right angle at center.
उत्तर
Consider circle with center ‘O’ and has two parallel tangents through A & B at ends of
diameter.
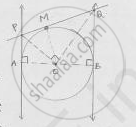
Let tangents through M intersects the tangents parallel at P and Q required to prove is that ∠POQ = 90°.
From fig. it is clear that ABQP is a quadrilateral
∠A + ∠B = 90° + 90° = 180° [At point of contact tangent & radius are perpendicular]
∠A + ∠B + ∠P + ∠Q = 360° [Angle sum property]
∠P + ∠Q = 360°−180° = 180° …..(i)
At P & Q ∠APO = ∠OPQ =1/2∠𝑃
∠BQO = ∠PQO =`1/2`∠𝑄 in (i)
2∠OPQ + 2 ∠PQO = 180°
∠OPQ + ∠PQO = 90° …. (ii)
In ΔOPQ, ∠OPQ + ∠PQO + ∠POQ = 180° [Angle sum property]
90° + ∠POQ = 180° [from (ii)]
∠POQ = 180° − 90° = 90°
∴ ∠POQ = 90°
APPEARS IN
संबंधित प्रश्न
In the given figure, PQ is a chord of length 8cm of a circle of radius 5cm. The tangents at P and Q intersect at a point T. Find the length TP

Fill in the blank
A continuous piece of a circle is ............... of the circle
Draw circle with diameter: 8.4 cm
In above case, measure the length of the radius of the circle drawn.
Draw circle with the radii given below.
3 cm
Two circles of radii 5 cm and 3 cm intersect at two points and the distance between their centres is 4 cm. Find the length of the common chord
The longest chord of a circle is __________
In the figure, O is the center of the circle. Line AQ is a tangent. If OP = 3, m(arc PM) = 120°, then find the length of AP.
In the following figure, BC is a diameter of the circle and ∠BAO = 60º. Then ∠ADC is equal to ______.
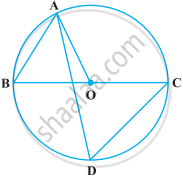
In the given figure, O is the centre of the circle. Name all radii of the circle.
A figure is in the form of rectangle PQRS having a semi-circle on side QR as shown in the figure. Determine the area of the plot.