Advertisements
Advertisements
प्रश्न
State, whether the pairs of triangles given in the following figures are congruent or not:
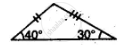
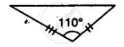
उत्तर
In the first A, third angle
= 180° – (40°+ 30°)
= 180° – 70°
= 110°
Now in these two triangles the sides and included angle of the one are equal to the corresponding sides and included angle.
Hence these are congruent triangles
(S.A.S. axiom)
APPEARS IN
संबंधित प्रश्न
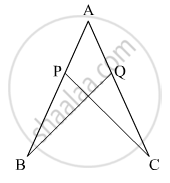
In the given figure, if AB = AC and ∠B = ∠C. Prove that BQ = CP.
In ΔPQR ≅ ΔEFD then ED =
If ABC and DEF are two triangles such that ΔABC \[\cong\] ΔFDE and AB = 5cm, ∠B = 40°
In the given figure, if AC is bisector of ∠BAD such that AB = 3 cm and AC = 5 cm, then CD =

In the following example, a pair of triangles is shown. Equal parts of triangle in each pair are marked with the same sign. Observe the figure and state the test by which the triangle in each pair are congruent.
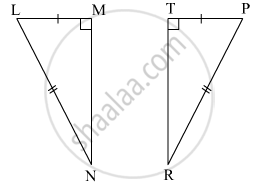
By ______ test
ΔLMN ≅ ΔPTR
If the following pair of the triangle is congruent? state the condition of congruency :
In ΔABC and ΔDEF, ∠B = ∠E = 90o; AC = DF and BC = EF.
The following figure has shown a triangle ABC in which AB = AC. M is a point on AB and N is a point on AC such that BM = CN.
Prove that: (i) AM = AN (ii) ΔAMC ≅ ΔANB

In ΔABC, AB = AC, BM and Cn are perpendiculars on AC and AB respectively. Prove that BM = CN.
Two right-angled triangles ABC and ADC have the same base AC. If BC = DC, prove that AC bisects ∠BCD.
In triangles ABC and PQR, ∠A = ∠Q and ∠B = ∠R. Which side of ∆PQR should be equal to side BC of ∆ABC so that the two triangles are congruent? Give reason for your answer.
